#Logic Circuit

[jbfly46]

Corporations sold the idea that artificial intelligence would remove the need to work to the general public. This will never be true. Currently, artificial intelligence is only being used by a small amount of people to do better work. Everyone else is being trained by the AI and not the other way around. In the end AI will only be used to verify if something is true or false. A basic LLM running on a quantum logic circuit.

[ctis2sherr]

https://www.futureelectronics.com/p/semiconductors--logic--74-series--a-hc-t/74hc594d-118-nexperia-1604081

74 A/HC/T Series Applications, programmable logic gate, digital gates

74HC Series 6 V 8-Bit Shift Register with Output Register - SOIC-16

[omne2ddy]

Bus-hold devices, 74HC family High-speed CMOS circuitry, Logic Circuit

74HC Series 6 V 3-State Surface Mount Shift Register - SOIC-16

[jhn2nberg]

Logic Circuit, Types of 74 A/HC/T Series Logic Chips

74HC Series 6 V 3-State Surface Mount Shift Register - SOIC-16

[mich2guezz]

74 A/HC/T Series Logic Chips, Types of 74 A/HC/T Series Logic Chips

74HC Series 6 V Dual Retriggerable Monostable Multivibrator with Reset - TSSOP16

[crpt2rciaa]

74 A/HC/T Series logic chips, Logic Circuit, digital gates, gate logic

74CBTLV Series 3.6V 4 Bit High Speed Bus Switch Surface Mount - TSSOP-14

[tequilazaku]

More like Quine McClussy method amirite!?

[homebrewstims]

Reila Audrey Haas / Logic

x x x x x x x x x Banner

[a-fox-studies]

Oct 29, 2023 • Sunday

GUESS WHO'S BACK

Exams are just around the corner, and I have recovered (mostly) so got back to productivity. I missed my notes <3

The 1989tv vault tracks are stuck in my headddddd my favourite is Now that we don't talk. If you are a swiftie, what is your favourite vault track?

Things I did today:

40 out of 55 slides of a PPT

19 out of 27 concepts from Module 1 of computer science

Ate biryani :3 (and then had a tummy ache LOL)

P.S. the whiteboard is @studaxy's I STOLE IT (jk, they gave it to me lol)

🎧 Suburban Legends — Taylor Swift

[chai-en-kaadhale]

a triangle and a logic gate enter a bar

((context under the cut if u dont get it))

this is an inverter (circuit/breadboard thing)

if you input something it'll output the opposite

in other words, input "A" and itll output "NOT A"

usually used for conditions like "f B and not A then C"

this is a sign that engineering is taking over my life

send help

[futurebird]

Using woodlouse logic gates in your natural circuits.

Isopods have 7 pairs of legs all pretty much the same length.

74** logic gates have … 7 pairs of legs all the same length!

Coincidence? I think not!

Clearly, each isopod contains 4 logic gates. This Armadillidium vulgare has 4 AND gates. Don't forget to connect power & ground legs & use pull-down resistors on inputs.

(best practice is to simply ask the rollie to position itself in your circuit.)

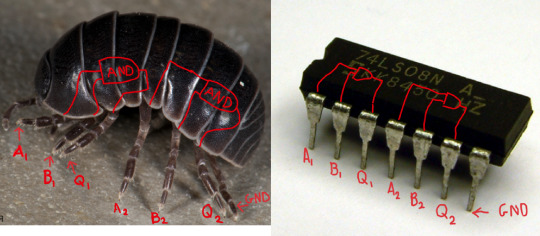
Like most natural systems. they use micro-voltages, don't use standard 5V power sources.

[lorillee]

GUYSSSSSSSSSSSS Its sojoever

[jhn2nberg]

Logic Circuit, Types of 74 A/HC/T Series Logic Chips

74HC Series 6 V 3-State Surface Mount Shift Register - SOIC-16

[fengshenjunlang]

Imagine judging mdzs using Christianity be like...🙄🙄🙄 even Buddhism could not be used to judge mdzs as literature. Why? Simply because XianXia literature isn't about religion! Religion is revolving around a God, whereas XianXia's concept is about "becoming a God themselves". The immortality concept in XianXia isn't about living forever to wait for true love like a certain vampire, however, it's about advancing to another higher realm and becoming Gods or Immortals.

The Lan Clan descended from a monk, but does any of the Lan Clan behave like a devoted monk? Of course not. Because they no longer are a monk. They're Daoist. Even Song Lan who came from a Temple, did he behave like a legit monk? Naturally not, because the Temple in mdzs has also been integrated with Daoism. The Buddhism in MDZS or XianXia literature is not the orthodox Buddhism as religion. It is a WAY, a PATH, to attain the Dao, in Daoism culture.

So, even using the related religion, Buddhism, couldn't be used to judge mdzs without considering its Daoism concept, not to mention using Christianity, a concept extremely out of context with the literature to begin with????

How to judge mdzs? Isn't it obvious?? Of course you have to use the Foundation of mdzs itself, a XianXia literature, with Daoism Concept, you dimwit!

[crpt2rciaa]

74 A/HC/T Series logic chips, Logic Circuit, digital gates, gate logic

74CBTLV Series 3.6V 4 Bit High Speed Bus Switch Surface Mount - TSSOP-14

[korpuskat]

Crying weeping sobbing NO don't do that to him he's my baby

What if Y/N got reborn. What then. Maybe as an omnic this time, or they stay human, either way they remember and they gotta kiss Ramatta

OR they don't remember, but Ramattra just knows it's them, their eyes, their demeanor,

"This isn't the first time we've met, is it?"

"No, my love"

"I'm glad you found me again"

"I never stopped looking for you, and even after the end, when the sky is burning, and this planet is no more, if you perish with it, I'll look for you."

"Will you find me?"

"Always"

"I'll meet you half way there"

"I'll be waiting"

This is so tangentially related but bear with me ok. What the fuck do we call Orisa and Echo? They aren't technically omnics (i.e. not built by Omnica corp nor gained sentience via the Awakening-- hell, the most recent Echo novel had Liao not entirely sure that Echo was sentient yet, right?)

But! Being reborn as some kind of synthetic life has so many interesting implications. Why did your soul get stuck in this body? Was it just luck that you get to go around again in a form more familiar to him or was there a reason? (and likewise, who made your body & for what purpose?)

I love that he would just... believe you. Just seeing someone and knowing it's you, not understanding how or why...