#mathematical functions

[er-cryptid]

Horizontal Translation of Logarithmic Functions

-- Formulae: -> g(x) = logb (x + c) -> g(x) = logb (x - c)

-- g(x) = logb (x + c) shifts the graph of f(x) = logb (x) to the left c units and moves the vertical asymptote to x = -c

-- g(x) = logb (x - c) shifts the graph of f(x) = logb (x) to the right c units and moves the vertical asymptote to x = c

.

Patreon

[arcsin27]

if youre bisexual youre also insexual and sursexual

[mentalknot]

It’s nights like these where I really miss playing Graphwar with the bros in 2021

my math career peaked in high school because that’s when I learned how to combine sine/cosine functions with absolutely everything for increased coverage, AND fine-tune my aim with absolute value functions…

10/10 my friend was absolutely destroyed… and then subsequently learned how to do 10x more damage after only a week!

[bismuth-soup]

Close up butt pics for relaxation and chakra healing

[xyymath]

Symmetry: The beauty of Mathematics

Listen, symmetry isn’t just aesthetic. It’s the backbone of mathematics, the reason your equations behave, and honestly, the only thing keeping some functions from spiraling into utter chaos. And here’s the truth: symmetry isn’t just about looking pretty; it’s about mathematical harmony, balance, and the deeply satisfying moment when everything aligns perfectly, just as it should.

The Y-Axis Mirror Selfie (a.k.a Even Functions)

2. The Rotational Drama Queens (a.k.a Symmetry about the Origin; odd functions)

3. Group Theory Symmetry : the reason your Rubik's Cube works and why physicists don’t spiral into existential despair when studying quantum particles.

Cyclic groups, Dihedral groups and Lie groups

4. Polar Coordinates—aka the Art Students of Math

Polar graphs SCREAM symmetry. Roses? Circles? LEMNISCATES?

the bottom line : Symmetry is how we recognize order in chaos. And symmetry isn’t just confined to math. You see it in biology (DNA), art (da Vinci’s Vitruvian Man), and even in the way we naturally find faces with symmetry more attractive. It's everywhere, from the humble parabola to the complex eigenfunctions of quantum systems. So next time you plot a graph, take a second to appreciate the sheer elegance of its symmetry. Or lack of symmetry—because asymmetry can be just as hot.

[elkement]

"Putting things in perspective..." ;-)

Next test in my project "Setting the Spherical Harmonic Functions free": freehand constructions using the techniques of traditional descriptive geometry, just without ruler or compass. Spherical harmonic function Y_32, as seen from a wild perspective! I am testing how to place the different views on the drawing plane as the view in perspective is so much smaller than the orthonormal views. Also playing with old-school lettering, inventing conventions for how I tag my points. My original inspirations to pick up a pen again have been antique patent drawings and Roger Penrose's hand-crafted drawings.

Graphite pencil / water color pencil / ballpoint pen / marker.

[lipshits-continuous]

I wonder if I could slip in some þ's into my project report

[wouldyoulikeafourleafclover]

Got my math test on functions back, just missed an A by about 3.5 marks so I am still on a B!

[gay-mathematician-portmanteau]

give me a harmonic function and i can find its conjugate no problem,,, but i can't help but wonder,,, when will i learn to make myself analytic :(

[evil-jennifer-hamilton-wb]

Y'all desmos has complex numbers now!!

(Complex numbers are just numbers to a girl like me, but big news for everybody else so I thought would disseminate.)

It was such a pain in the ass to do complex functions in desmos before, I have spent so many hours trying to get real number based desmos to do domain coloring with complex functions. I haven't actually had a chance to try it out yet so I don't know exactly how it's implemented.

(we did the same thing before they added 3D where you could use parametrics in 2D to graph any 3D explicit function, but it was a pain and took a long time to figure out how to graph 3d on a 2d space. (It took a while to engineer for me at least, idk if it was actually easy and I'm just stupid))

[jucomx]

Surprising absolutely noone except undergraduate math students, it turns out math actually is only about numbers in the end!

[eulerseverything]

About to start a new semester, taking Foundations for Theoretical Mathematics and Differential Equations. Very excited to become even more annoying about math to my friends. Anyway does anyone on mathblr recommendations for either course cause I’m a freshman taking upper divs so I need the help

[daily-public-domain]

Day 247: Riemann Zeta landscape

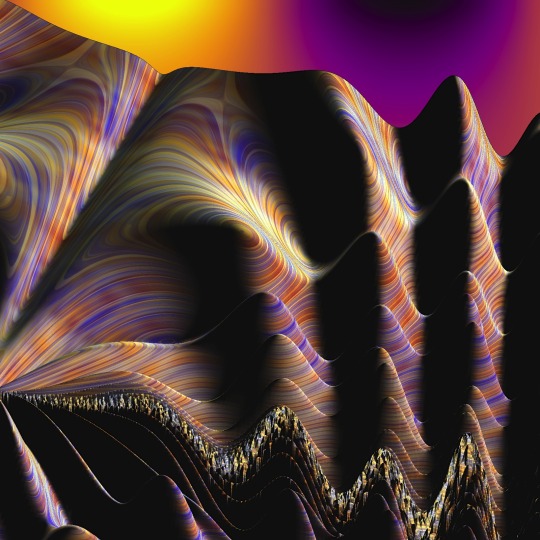
link

–This image is part of the public domain, meaning you can do anything you want with it! (you could even sell it as a shirt, poster or whatever, no need to credit it!)–

[a-fox-studies]

August 9, 2023

Learning in the dark has a completely different vibe.

🎧 Sheltered - Cauzy

[art-of-mathematics]

Silly captions only please.

[elkement]

elkement is featured at Mathematical Art Digital Exhibition!!

... and I still cannot believe it!! I am over the moon and still do not believe it!

Artworks of my 2023 series Reality and Imagination are part of the Mathematical Art Digital Exhibition at Queens College, New York!!

Images are now shown on screens in the new multimedia space at the math department and online at:

https://www.qc.cuny.edu/academics/math/made-gallery/

This is the first time ever my art is "officially presented" - in a place other than my website or social media! I am over the moon!

Thanks to everybody who reposted or liked my art! You have given me the courage to submit my art! I am also grateful that the curator of the exhibition, math professor and math artist Christopher Hanusa created a truly open call for art - not requiring a formal education in art or a track record as an artist!

I've submitted four images out of thirteen in the series to the exhibition. Here is the complete series on my website, with more background and links to the many different artistic experiments I did with Reality and Imagination - real and imaginary part of complex function 1/z :-)

All thirteen images are available for sale as prints on INPRNT - as fine art prints, posters, stickers, acrylic/metal/canvas prints, art cards, mini prints.

Here are the ones in the exhibition, each shown with a different type of print:

Reality and Imagination #3 - link for fine art print:

Reality and Imagination #5 - showing the art card version:

Reality and Imagination #0 - e.g. available as acrylic print:

Reality and Imagination #12 - as a cute mini print: