#mathematical proof

[secondwheel]

Proof in maths

[kree8r0]

Why do people ignore the obvious proof of God?

This post is a response to a question posed in its complete format: “How come people ignore the mathematical proof of God, even when it is so obvious? How did humanity convince itself that the One cannot be proved mathematically?” A general rule of thumb is when something seems “so obvious” to you, but the rest of the world fails to see what you see, it is incumbent upon you to do what you can…

[simplicius-simplicissimus]

Q.E.D. - quod erat demonstrandum (that which was to be demonstrated)

-Placed normally at the end of mathematical proofs or philosophical arguments

[catscomputerscreativewriting]

It's so funny to me that people think of Math/Mathematicians as being hyper-logical and rational. Like, have you seen some of the wild things hiding in the Math?

Did you know there are non-computable numbers?? (https://en.wikipedia.org/wiki/Chaitin%27s_constant)

Did you know that there are things that are true, but we can't prove them??? (https://en.wikipedia.org/wiki/G%C3%B6del%27s_incompleteness_theorems)

Did you know that we can prove that something exists, and yet never actually figure out what that thing is?? (https://mathworld.wolfram.com/NonconstructiveProof.html)

Math is crazy. Math is wild. Math hardly makes sense, and when you think you understand the weirdest parts of it, everyone who hears you explain it to thinks you're a gibbering lunatic.

"In mathematics you don’t understand things. You just get used to them." - von Neumann

(please share more unhinged math with me, i want to see more scary math)

[mentalknot]

As a math major trying not to get bogged down in the hellscape of severe social anxiety once again, proofs are shockingly comforting.

How wonderful to behold a carefully chosen sequence of arguments and accompanying symbols that remains absolutely logical…

…while navigating a mind that does nothing but lie at every juncture of my life.

[duskofastraeus]

Exam Season Diaries 2

- Finished revising for my Differential Equations Exam

- Completed my Differential Equations Methods Exam (Survived (sort of)/Did not Survive)

- Re-did all Thermodynamics problem sheets

- Re-did all Statistical Mechanics assignments

- Revised all Thermo material

Notes of the day:

What in the actual hell was that exam.

[catboydan]

my very specific prediction for the upcoming AITA video: he'll open by explaining that last week on the gaming channel he had an 'AITA' moment himself [insert clips from the dan eye incident here]

then he'll lead into the vid by saying something like "and that inspired me to ask you all for your 'am I the hole' moments", and only then will he bring up that this time, he asked for specifically dating/love-related stories, leaving us to piece together that the intro is a dating/love related story via the maxim of relevance

[pv1isalsoimportant]

anime girl proof complexity! ANIME GIRL PROOF COMPLEXITY!

Logic seminar at IM CAS; Metamathematics of Resolution Lower Bounds: A TFNP Perspective, Hanlin Ren, Oxford Univ.

[shadowyyyidk]

Isn't the Penrose tiling just an absolutely beautiful concept? The same bunch of shapes are being used, and similar patterns are being formed at multiple places, and yet they are never repeating, not even once, because of an infinite number of conformations.

[cams-math-rants]

Intro to Analysis

I've started studying real analysis over the summer since it is a class I will be taking next semester, and I am greatly enjoying it so far. I would like to share an interesting proof I read about.

The proof I am showing is that ℚ is dense in ℝ:

(a) x,y∈ℝ and x<y ⇒ ∃p∈ℚ such that x<p<y

Which means for every two real numbers x,y where x<y, you can always find a rational number between them.

To understand this proof, you need to know about the Archimedean property:

(b) x,y∈ℝ and x>0 ⇒ ∃n∈ℤ+ such that nx>y

-----------------------

PROOF:

Since x<y, this implies that 0<y-x. By (b), we see that ∃n∈ℤ+ such that (1/n)<y-x, or that 1<n(y-x)=ny-nx.

When applying (b) again, we have that ∃m1,m2∈ℤ+ such that m1>nx and m2>-nx. Then, we get -m2<nx<m1.

We know that ∃m∈ℤ, where m2≤m≤m1, such that m-1≤nx<m.

Then, nx<m≤nx+1.

Since 1<ny-nx implies ny>nx+1, we have that nx<m≤nx+1<ny.

Since n>0, it follows that x<(m/n)<y.

This proves (a) for p=(m/n).

∎

Sources:

Rudin, Walter. Principles of Mathematical Analysis. 1953. 3rd ed., ‎McGraw-Hill Publishing Company.

The song I'm currently listening to:

[simplydnp]

who's hand is in this picture?

A Mathematically Rigorous Proof That I Spent Too Long Writing

welcome to university math: dnp hand edition

(no, don't leave, you'll be fine i promise)

to begin, we need a statement to prove. we have two options:

- the hand is dan's hand

- the hand is phil's hand

now, for most proofs in university math, you are told a true statement, and you must show why it is true using logic rules, definitions, and theorems. but, we do not know which of these statements are true, so we have to find out.

to prove that a statement is true, we must show that it is always true for the situation presented. to show a statement is false, we must present a single instance where the statement is false (also known as a counter example).

a quick not scary math example:

definition: a prime number is only divisible by 1 and itself.

statement: all prime numbers are odd

(this is false, because 2 is a prime number and it is even. you don't even need to check if there's any others, all you need is one single case where it isn't true to disprove it)

so now that we have a little background on proofs and how to prove and disprove them, we go back to our two statements.

the thing with this situation is, one of them must be true (unless you're gung-ho on someone else holding dan's face while phil takes a picture on his phone of dan in his glasses, in which case, i applaud your commitment, but in actuality this proof will cover that option too)

the full statement we have is: dan is touching his face or phil is touching dan's face

now, because this is Real Life and we have a picture where a hand is touching dan's face, we know already that one of these options is true (as mentioned above) but! using symbolic logic you could also come to this conclusion.

this type of statement is an 'or' statement, and if you're curious, you can look into 'truth-tables' and see why, but at least one of the options must be true.

back to the proof at hand (bah-duhm-tss)

okay. now, proofs also must be 'general' in order to mean anything, really. these are statements of truth of the universe, not just for individuals. so, we will prove this generally.

we have 2 people involved, so individual 1 (dan, the owner of the face and potential face toucher) will be labelled as 'D' , and individual 2 (phil, the possible face toucher who does not own the face) will be labelled as 'P'. thus, this can be true for any such D and any such P.

so with our 'or' statement, in order to prove it, we pick one of the options and say that it is not true, and we have to show then that the other is true.

step 1: let's assume this is not P's hand. (assumption)

step 2: thus, it must be D's hand. (what we take from our assumption)

step 3: now, if it is D's hand, we look at what a hand on one's own face is capable of appearing like. (a definition or true fact about step 2)

the position in the given photo shows the hand with a thumb on the cheek, and a finger on the forehead. so, we find an example of a person with their fingers in the same position (or close to) and see if this supports our claim.

consider:

now, with this image, you can clearly see how the subject's right hand has the thumb on the temple and index finger on the top of their head, however, it is a close enough position for our case.

from the view of the camera, the closest finger to the camera is the edge of the pinkie. in fact, it will always be the closest finger to the camera in this position, assuming the subject has all fingers and no additional appendages.

step 4: we now compare this to our photo (we verify if this holds to our claim or contradicts it)

in our photo, the closest appendage to the camera is the edge of the thumb.

step 5: thus, it cannot be the case that D is touching their own face. (what the evidence says)

step 6: as we assumed it was not P's hand and have shown it cannot be D's hand, and as this is an 'or' statement both of these claims cannot be false, we can therefore conclude it must be P's hand. (our conclusion: re-stating the statement and assumptions and conclusion)

step 7: we verify that P is true (optional step but in beginner proofs you generally show why your case works)

to do this, i will show a picture of a person touching another's face, and compare it to our image.

consider:

now, this image is not exactly the same, similar to above. however, P's left thumb is on the cheek, with their index on D's temple. the closest appendage to the camera (if it were in a similar perspective as our original) would be the edge of the thumb.

comparing it to our original:

our comparison holds.

thus, we can conclude that the true claim in this statement is that P must be touching D's face, which, in particular means that:

phil is touching dan's face in the image

thank you for partaking in phannie mathematics. we now know. i am not sorry.

bonus:

phil has a hitchhikers thumb and dan doesn't so why was this necessary at all 🤡

[exnihilo-comic]

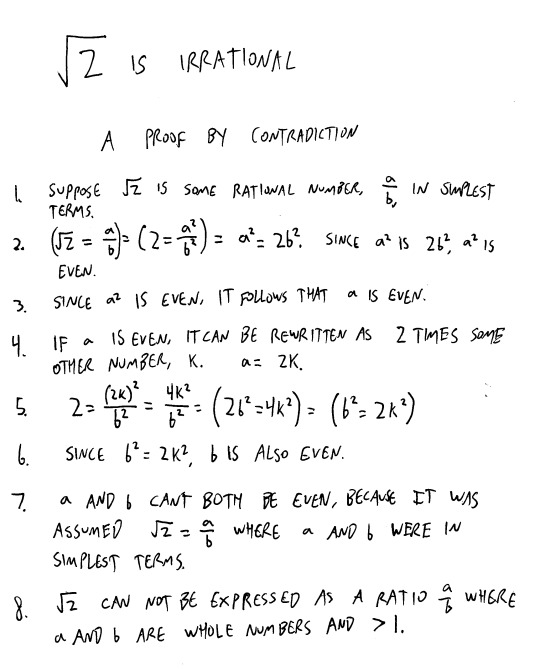
it was late and i didnt feel like drawing anymore so i wrote out one of my favorite proofs

[roariescorner]

Omg is that a vocaloid reference?!?!?!?!!?!?

PERPENDICULAR BISECTING LINES?? GEOMETRY TIME!!!

Click!

[mentalknot]

Sharing of Proof Between Friends

After spending over nearly eight hours of each day in a mathematics department for two years straight, I’m shocked that many high schoolers believe math is a solitary pursuit.

In reality, this community seems to be one of most welcoming and collaborative academic communities I’ve found. Let me share some moments:

“Hey! How’s it going?”

“Alright, been stuck on this interesting question my friend emailed me the other day… he said the first part of the proof is pretty easy, but I’ve been at it for 10 hours…”

“Never trust a mathematician who throws around the word ‘easy,’ c’mon let’s try it together at the board.”

Thirty minutes later, they had completed the proof, and sat back with wide smiles to admire their work. In truth, there was rarely a conversation that didn’t eventually turn to math in that department…

“Yeah, and I heard that the guy cheated with his best friend’s sister… wild right?”

“Yeah… not to interject, but I have this representation theory question… would you all be willing to take a look?”

The conversation took an immediate turn with collective enthusiasm. I have been lucky to have my own “collaborative math” moments since returning to my undergraduate studies, and do my best to share this part of “math culture” with younger students curious about the major.

“So that’s the proof that motivates our paper! It’s quite short, but there’s something about it I love.”

“Wait… but you’ve only done half, and this is a biconditional statement, let’s try it together.”

There was a reason my mentor never encouraged me to look at the other side of the proof… it was far more “ugly,” but tons of fun to piece together with a fiend. We looked back at our work after forty minutes with satisfaction before returning to our neglected problem sets…

And finally, I tried to assist a student with a calculus question using the “process of questioning” the research world had taught me:

“I need to find a closed-form equation for this geometric series… but I can’t seem to get the alteration sign?”

“Try writing out the first six terms, do you see anything that you could simplify? Look at the denominator specifically…”

“Well, they’re all multiples of three…”

“Try pulling that three out, any more similarities?”

“The numbers multiplying the threes are powers of two! But I still need that alternating sign…”

“Remind me, what happens when you raise a negative number to an odd/even power? Try it with (-1)^n”

“If it’s odd, the number stays negative, and positive if even… so if I add this to the denominator, the sign is alternating depending on the index n!”

“YES! This little (-1)^n trick comes up everywhere, it’s a nice ‘tool’ to hold on to if you decide to take more math.”

The exchange was wonderful… and motivated me to review the calculus I’d excitedly ran past when I was younger. I wish this type of discourse was taught more expansively.

[marigold-22]

Contrapositive are the same as contradiction, they shouldn’t have different names

“Suppose A, now for the sake of contradiction suppose -b then… -A, a contradiction, thus A implies b.”

Is the same thing as

“Suppose -b, then… -A. Therefore by contrapositive A implies b.”

Their just linguistically different but that doesn’t make them different, anything provable with the first technique is provable with the second technique and vice versa. Contrapositive adds nothing.

[noosphe-re]

In 1931, the Austrian logician Kurt Gödel pulled off arguably one of the most stunning intellectual achievements in history. Mathematicians of the era sought a solid foundation for mathematics: a set of basic mathematical facts, or axioms, that was both consistent — never leading to contradictions — and complete, serving as the building blocks of all mathematical truths. But Gödel’s shocking incompleteness theorems, published when he was just 25, crushed that dream. He proved that any set of axioms you could posit as a possible foundation for math will inevitably be incomplete; there will always be true facts about numbers that cannot be proved by those axioms. He also showed that no candidate set of axioms can ever prove its own consistency. His incompleteness theorems meant there can be no mathematical theory of everything, no unification of what’s provable and what’s true. What mathematicians can prove depends on their starting assumptions, not on any fundamental ground truth from which all answers spring.

Natalie Wolchover, How Gödel’s Proof Works, Quanta Magazine, July 14, 2020