#James Tanton

[willstafford]

Ghost With The Most

PHANTOM OF THE OPERA The Attic Theatre, Stratford upon Avon, Wednesday 23rd October 2024 Not the overblown Lloyd Webber spectacle, this is a new adaptation of the Gaston Leroux story by Tread The Boards’ writer-in-chief, Catherine Prout – the same writer who has tickled us pink with comedic versions of other classics.  Here, she dispenses with the knockabout humour in favour of melodrama.  The…

[drberkes]

Hang in there

Matthew's Jounal 5/25/24

"When you reach the end of your rope, tie a knot in it and hang on." - FDR

I want to take the time to thank everyone who helped me get to where I am today. The meaning, joy, hope and purpose I found because of the legendary people I got to work with and learn from is more than enough.

Aside from connecting with people and making a difference however I can, what is most important to me is not letting ignorance win. Identifying ignorance as the source of all the pain I've experienced or caused in my life is comforting because it makes the theme of my long-term goals clear to even myself. What did I learn and who did it help? Even if this is too simplistic, it'll do well enough for now.

"Love is enough, it always is," was one of the things I said in group during my hospital stay in 2022. I want to walk that back today. I think it was SIgmund Freud who said "Love and work, that's all there is." This is better.

Anyway, in case you didn't know, the reason I was at the hospital then is because I made a series of mistakes. I'm sorry. "For all sad words of tongue and pen, the saddest are these, 'It might have been.'” – John Greenleaf Whittier

Part of living my life has necessitated not giving up. I'm hoping to get back into classes this Summer or Fall and keep hanging out in the behavioral heath field for a while. I'm not sure when I'll finish my math degree, but it remains a goal of mine. To steal from James Tanton, in math it sometimes seems like we're only taught the grammar of math: how to do the problems. I fully believe that there is poetry in math and I want to unlock that joy for myself and the people in my life. Winston Churchill has my back on this one.

Best wishes

[mirandamckenni1]

Liked on YouTube: The Strange Number System Where Infinity Is Tiny || https://www.youtube.com/watch?v=tRaq4aYPzCc || There's a strange number system, featured in the work of a dozen Fields Medalists, that helps solve problems that are intractable with real numbers. Head to https://ift.tt/YhHbl3X to start your free 30-day trial, and the first 200 people get 20% off an annual premium subscription. If you're looking for a molecular modeling kit, try Snatoms - a kit I invented where the atoms snap together magnetically: https://snatoms.com ▀▀▀ References: Koblitz, N. (2012). p-adic Numbers, p-adic Analysis, and Zeta-Functions (Vol. 58). Springer Science & Business Media. Amazing intro to p-adic numbers here: https://youtu.be/3gyHKCDq1YA Excellent series on p-adic numbers: https://youtu.be/VTtBDSWR1Ac Great videos by James Tanton: @JamesTantonMath ▀▀▀ Special thanks to our Patreon supporters: Emil Abu Milad, Tj Steyn, meg noah, Bernard McGee, KeyWestr, Amadeo Bee, TTST, Balkrishna Heroor, John H. Austin, Jr., john kiehl, Anton Ragin, Diffbot, Gnare, Dave Kircher, Burt Humburg, Blake Byers, Evgeny Skvortsov, Meekay, Bill Linder, Paul Peijzel, Josh Hibschman, Mac Malkawi, Juan Benet, Ubiquity Ventures, Richard Sundvall, Lee Redden, Stephen Wilcox, Marinus Kuivenhoven, Michael Krugman, Sam Lutfi. ▀▀▀ Written by Derek Muller and Alex Kontorovich Edited by Trenton Oliver Animated by Mike Radjabov, Ivy Tello, Fabio Albertelli and Jakub Misiek Filmed by Derek Muller Additional video/photos supplied by Getty Images & Pond5 Music from Epidemic Sound & Jonny Hyman Produced by Derek Muller, Petr Lebedev, & Emily Zhang

[444names]

the list of every x-men ever from comicvine

Actan Actarbes Affes Affining Aptimis Aptinet Aubitch Avilkett Backe Baing Barded Bardt Barned Barry Barser Barza Becon Becot Becotter Behilan Behitt Bernto Blibs Bline Blonso Blower Blowle Bolashan Boodanc Booder Boodged Booratims Boorm Boors Bowns Braunded Braynet Briers Bries Briors Briott Broratons Broshilly Bucens Buchmas Buckeror Buckining Buckwer Burch Bureassal Bureques Burgester Burna Burry Cable Cadis Caing Caletchno Camers Camone Candez Caned Carle Carmed Carrever Carrielps Carve Cased Caspenn Casper Casso Caste Cater Caterter Challion Chalt Chamed Chater Chatina Chation Chayne Chewiste Chnetch Ciner Cland Clanfient Clastiong Clawlan Cle's Cloperann Clugher Clured Cocks Comenry Coment Comerner Comerrior Cookes Coort Cosed Coshaman Costia Cought Coylon Cruilke Crusense Crushamed Cruturn Curgesis Cyced Cycon Cyconsh Cycott Dalled Danch Darko Darrining Dencon Dennetch Derreganc Disalton Disolo Donobb Dunkesell Dunks Eadmic Eldesing Elson Evelash Everte Exilarer Exill Exillorn Fackley Fadons Fajarlo Fantought Firber Firly Fised Fisone Forge Forned Frader Fratts Friffray Frine Fught Gairlds Garby Garcall Garion Garly Gilkessus Gilknolis Ginest Ginine Goranne Graters Grinly Griolive Grionson Guell Guild Guitions Haark Handax Hantrobb Hapmayne Harez Harmance Harto Heralen Herecomem Heroes Herry Hicashook Hogarse Hoginamb Hostentop Hullichne Hunce Hundefley Indeash Ininley Jamann Jamed Jardt Jillibs Johne Joider Joirly Kautorto Kersonord Kniffes Kniguyed Knine Laccale's Lains Lated Laten Leally Leance Leaned Leark Leatfice Leaucks Leautly Legass Legates Letch Letchip Lettevy Letudle Levarre Llann Lomen Lomey Lomider Loppoine Lopted Lossus Lostes Maccasend Mackwerse Madistard Manets Manton Marko Marld Marmagne Marts Mashirde Mccarson Mccrus Mclono Mclonsons Mcloodax Mclugshew Mcmidd Mcmidy Mcminn Mcmins Merry Merts Mesin Messanton Mindy Mojohen Molowns Mooden Moodged Morged Moriffe's Morress Mortese Mostin Moston Muirby Muird Muirdy Muitaine Munks Munkso Mutory N'gabect Nallever Nally Niguarver Nigues Niguirle Niguyed Niguyes Niono Oblis Orairly Orawley Orawyed Orayed Orged Pareans Paric Parko Parton Parvans Patfich Pating Pation Patlyne Patts Penter Phries Phriffega Phring Phrion Poine Poinly Posalanne Posminsee Posson Postainn Poster Posters Powmanes Prides Printor Proacks Probb Proes Prord Prorparm Prosmin Pullshays Raing Rainges Randere Retchamon Retevest Retudley Ricarrez Rions Rodgenn Rodulds Rolackint Rosan Rosanday Rossalent Rossaver Rostions Rought Rournet Routhcre Rovestan Ruirks Ryoudlest Ryoundes Sacke Sackmate Sagned Sally Santom Saves Savider Scile Sellon Shaylowle Sheraing Sherse Shewiller Shirks Shitanfic Shiverr Skrutlee Smalis Sonobs Spitt Stanker Stann Stardisol Starrett Starry Sterega Stilch Stild Stomey Stonce Stralds Surankes Surch Sureanson Surerry Surmagare Syleal Tanced Tanton Tesens Testsoner Theevy Thraut Torne Torternt Tortorry Trobster Tromem Tromer Trosboon Trostes Trostordt Turez Turging Turreeve Tuser Twister Undayed Unded Under Unobstion Unolon Updax Updayed Updays Valvany Vandleyos Vansfoll Virdt Vires Virle's Virly Virobb Vouggenn Wadent Wading Wadmis Walle Wallop Warchway Wartilker Wellic Welps Wersons Whent Whews Whewson Whewsonts Whiley Whitar Willons Winamer Wittes Woliancip Wolon Wooden Worguabre Worman Xavarby Xaveallso Xavok Xavourna Xi'an Xi'aning Xi'anson Yalan Yalinn Yalleray

[elisabethloxx]

Jayne MacDonald, aged 16, a shop assistant who had recently left school, was the first non-prostitute, or an "innocent victim" as it was proclaimed, to die by the hand of Peter Sutcliffe, the Yorkshire Ripper.

On Saturday, June 25th, Jayne MacDonald had gone to meet friends near the Leeds city centre at the Hofbrauhaus, a German-style 'Bierkeller'. There she had met an 18-year-old named Mark Jones, with whom she had danced with. At 10:30 pm, the two set off, as part of a crowd, in the direction of Briggate, the main shopping street. Jayne had suggested going for chips and by the time they found a place, bought and eaten them, Jayne had missed her last bus. They sat on a bench until about midnight, before walking towards where Mark lived on an estate near St. James hospital. Mark told Jayne that if his sister was home then she could give Jayne a ride home. When it was obvious that his sister was not home, her car not being outside the house, they continued by his house and up Beckett Road in the direction of Chapeltown, and Jayne's home. They lay in a field for a while until after 1:00 am.

The two parted company outside the main gates of the hospital at around 1:30 am. They had agreed to met again later in the middle of the week. Jayne's intention was to call a taxi from the taxi firm's kiosk at the corner of Harehills Road. However, when she received no reply at the kiosk, she decided to continue on walking and came out of the maze of streets near the Grandways supermarket, were she worked. She continued past the Gaiety, where Emily Jackson was last seen, and was walking along Chapeltown Road in the direction of Reginald Street, and her home at 77 Scott Hall Avenue, just six doors away from the home of the first Yorkshire Ripper murder victim, Wilma McCann.

Peter Sutcliffe spent the night of June 25th in the company of Ronald and David Barker, who lived on the same street where Peter and his wife Sonia lived with her parents. After a night of drinking in the pubs of Bradford, Peter deposited them at the end of Tanton Crescent, Clayton, Bradford, and, instead of going home himself, he turned the car around and headed for his "hunting ground" in Leeds.

At around 2:00 am, Peter Sutcliffe saw Jayne MacDonald walking on Chapeltown Road. He parked and watched her for a few minutes before getting out of the car after arming himself with a hammer and a kitchen knife from under his seat and putting them in his pocket. He was quite certain she was a prostitute. Not only was she walking in a red-light area late at night, but he claims he saw her stop and talk to a couple of girls on a street corner. He then began to follow her for a short distance, and she never looked around as he followed, even though the distance between them wasn't great.

About 30 yards into Reginald Street, near an adventure playground, Sutcliffe struck Jayne MacDonald with the hammer on the back of the head. After she fell down, he then dragged her, face down, about 20 yards into the corner of the play area. Her shoes made a "horrible scraping noise" along the ground as he dragged her. He hit her again with the hammer and then pulled her clothes up and stabbed her several times in the chest and in the back.

At 9:45 am two children made their way into the adventure playground between Reginald Street and Reginald Terrace and discovered the body of Jayne MacDonald near a wall. Spots of blood on the pavement at the entrance of the adventure playground quickly established where she had first been attack. Her body was found lying face down, her gingham skirt disarranged, and her blue and white halter-neck sun top pulled up to expose her middle. She had been hit on the head three times with a hammer and had been stabbed about twenty times in the chest and on the back. There was repeated stabbing through one wound in her chest and and one wound in her back. Blood smears on her back revealed that Sutcliffe had tried to wiped his knife clean. When the police turned over her body, they discovered a broken bottle with the screw-top still attached had been embedded into her chest. Sutcliffe would later claim that he did not deliberately embed the bottle into her chest, and that it must have happened as he dragged her through the rubble on the playground. A post-mortem examination showed she had not been drinking, but had only had soft drinks that night.

The slaying of a young girl, not connected to the prostitute trade, an "innocent", brought not only national attention to the case, and outrage from the public not seen in the earlier murder cases, but also caused Chief Constable Ronald Gregory to appoint his most senior detective, Assistant Chief Constable George Oldfield to be in overall charge of the escalating Ripper murder investigations. Peter Sutcliffe claims to have been shocked when he saw the newspaper headlines that Jayne MacDonald had not been a prostitute as he had assumed.

Jayne’s father, Wilf MacDonald, a former railwayman, was to die two years after her murder, never having recovered from the ordeal of her murder.

[mathhombre]

Polygon in Polygon

James Tanton, inspired by Vincent Pantaloni, tweeted an image I misunderstood, then found something cool. He shared the top image and I looked at it for a hot second and had to make it. Assuming that the letters referred to area. That worked for 6, 8, 10, ... sides, but not 4. James clarified he meant edges, which I obviously should have inferred, and was quick to verify. But, it leaves me wondering why the area case is different for n=4. 

The original Tanton tweet. The GeoGebra for play.

[ebouks]

8 Tips To Conquer Any Problem: Solutions Manual

8 Tips To Conquer Any Problem: Solutions Manual

8 Tips To Conquer Any Problem: Solutions Manual Tanton, James Categories: Education Studies & Teaching Year: 2016 Publisher: Edfinity Language: english Pages: 108 ISBN 10: 1944931015 ISBN 13: 9781944931018 File: 84 MB

[libr-tumbl-alternative]

A few months from now, if James Tanton and his Global Math Project co-conspirators have their way, ten million schoolchildren will take a huge mathematical step from the twenty-first century all th…. Powered by AutoBlogger.co

[perfectirishgifts]

The Best Way To Teach Math

New Post has been published on https://perfectirishgifts.com/the-best-way-to-teach-math/

The Best Way To Teach Math

Fractals are enjoyed by both mathematicians and artists.

Mathematics permeates all aspects of everyday life, from genetic code to GPS satellites. However, traditional math education often fails to instill a love for mathematics and habits of mathematical thinking in its pupils. We have gotten accustomed to seeing math education as a lifeless, impassive pursuit. Students are often frightened, traumatized and turned off by their math learning experience, while parents accept their children’s struggles as a given. 

Meanwhile, the 21st-century workplace demands competent problem solvers ready to take intellectual risks, try new strategies, and enjoy the challenges of the unknown. The educational approaches that seemed to work fine a mere half-century ago may no longer serve us well. Instead of well-trained calculators, we need people who know how to pose questions, how to deal with being stuck, and how to have enough self-confidence to look for new solutions. 

One of the most promising ways of encouraging students to study math is to put them in charge of their learning experience. Developmental psychologists and business leaders know that taking ownership of an activity fosters happiness and raises motivation. Students become real mathematicians when they create their own mathematical worlds that are intriguing to them. That’s why the motto of Maria Droujkova, math education researcher and the founder of Natural Math, a mathematical community for parents and children, is “Make math your own to make your own math!” 

Mathematics, by its very nature, is a creative endeavor. No amount of standardized testing can capture its infinite variety. If you ask a mathematician about the first words that come to mind when they think about mathematics, they will often mention beauty, open-mindedness and joy – not numbers or formulas. 

“The first thing to understand is that mathematics is an art,” says mathematician and teacher Paul Lockhart, the author of the Mathematician’s Lament – a paper, widely circulated among math teachers, and one of the most eloquent critiques of modern math education. Likewise, more than a half-century earlier, philosopher Bertrand Russell observed, “The pure mathematician, like the musician, is a free creator of his world of ordered beauty”. Creating your own math, like your own artwork, can be a great source of joy for all ages, and even some toddlers have tried their hand in it, building new shapes out of sticks, making color patterns and inventing their own dance routines.

At its core, math education doesn’t need to be about tests. The pandemic has (quite literally) brought this message home for educators. This past spring, many teachers around the country were not required to “teach to the test.” The change led many of them to look up the rich array of alternative approaches to math education, such as hands-on projects, games, coding and art. 

One such collection is the Global Math Project, a worldwide movement focused on joyful math experiences founded by mathematician James Tanton. Just over the past year, more than six million students and teachers from the US to Serbia to Tanzania took part in the Project’s  Exploding Dots Challenge, an uplifting mathematical activity that requires nothing more than pebbles or cheerios yet takes students all the way from kindergarten arithmetic to high school algebra.

Engaging mathematical explorations can help students overcome boredom and math anxiety by letting them enjoy the discovery of elegant solutions to seemingly intractable problems. What could be a better approach to developing a growth mindset than letting children experience the awesome powers of their minds firsthand? Let’s hope that “joy” will soon become the buzzword of math education.

From Education in Perfectirishgifts

[scifigeneration]

Magnetic memories of a metal world

4.5 billion years ago in the violent, high-speed environment of the early solar system, a protoplanet roughly the size of Mars was involved in a series of fierce collisions with other large planetary bodies.

A number of powerful impacts stripped the planet of its rocky mantle, leaving an exposed nickel-iron core. After further blows, break-away pieces of metal were flung into space -- the final destination for some would be Earth.

This, scientists believe, is the story of Psyche. Now a cold metal world in the asteroid belt between Mars and Jupiter, the asteroid -- named after the Greek goddess of the soul- offers a unique vision of the violent history of collisions and accretion that created the terrestrial planets.

Research published in Earth and Planetary Science Letters has helped secure $450 million of NASA funding for a satellite to boldly go where no satellite has been before; on a mission to Psyche.

The study, led by a Fellow at St John's College, Cambridge, Dr James Bryson, reveals the hidden magnetic messages in a rare group of metal meteorites which scientists believe are from Psyche. The results indicate that Psyche had a strong and unstable magnetic field and support the hypothesis that the asteroid is an exposed planetary core that cooled quickly due to the absence of a rocky mantle. These conclusions about Psyche, reached in the laboratory, will now be tested by an eponymous mission to outer space.

The Psyche satellite will orbit the asteroid while gathering data. If proved correct, a pan-institutional team of scientists led by Professor Lindy Elkins-Tanton from Arizona State University, will have the opportunity to directly measure a metallic core like the ones that lie at inaccessible depths and conditions within the terrestrial planets, including Earth. Exploring this unknown world will provide researchers with a remarkable insight into the building blocks of planet formation and will enable scientists and the public to see, for the first time, a world made of metal rather than ice, rock or gas.

The study of magnetic messages in meteorites can yield important information about their parent body. Like a hard drive, meteorites store magnetic information and can reveal whether the object they came from generated a magnetic field and if so what that field was like.

In the case of Psyche, finding out about the magnetic field it once generated would allow researchers to infer important conclusions about its properties and formation such as how and at what speed it cooled and solidified.

The clues contained within metal-rich meteorites, however, were thought to be impossible to decipher. This is because metal meteorites are primarily made out of large pieces of iron -- a material with a poor magnetic memory that engineers would steer well clear of when building a computer.

This changed when during his PhD, Bryson developed a pioneering detailed imaging technique to read the hidden magnetic memory of metal meteorites. "After developing the technique, I was talking about my work at a conference last year when I was approached by members of the Psyche team. They had a group of meteorites thought to be from Psyche due to their elemental composition and the rate at which they had cooled. They asked me to analyse them in the lab to see what they could tell us about the asteroid," said Bryson.

Hidden within the iron bulk of the meteorites, Bryson identified tiny particles of a metal called tetrataenite. This material is magnetically much more stable than iron and is capable of holding a magnetic memory going back billions of years. Reading the tetrataenite was a challenge because the particles measured between 10-100 nanometres (one nanometre is one billionth of a metre). However, using a big ring of magnets called a synchrotron which fires electrons around at near the speed of light, Bryson was able to produce intense beams of x-rays that allowed him to image the particles and measure the nanoscale magnetisation of the meteoric metal.

In addition, Bryson then carried out more conventional measurements of the magnetism of tiny pieces of rock contained within some of the meteorites using a magnetometer.

The results were consistent with the theory that Psyche is an exposed metal core as they suggest that the asteroid cooled very quickly, something scientists would expect to observe in a core stripped of its protective mantel. The meteorites contained the memory of an intense magnetic field, far stronger than Earth's. Planetary magnetic fields are produced by the churning motions of liquid metals in a planet's core that conduct electricity and have an electrical charge. Faster cooling generates strong convection currents which drive the liquid core to swirl faster and produce a more powerful magnetic field.

The secrets given up by the meteorites also suggested that Psyche's magnetic field was volatile, another property consistent with rapid cooling. When there is a vigorous liquid metal motion in a core caused by rapid cooling the positions of magnetic north and magnetic south are unstable and can interchange. The meteorites contained a record of being magnetised in different directions at distinct times supporting the idea that Psyche's magnetic field alternated in its polarity.

Evidence from the meteorites also indicates that, unlike the cooling process currently underway in Earth's core, Psyche cooled from the outside in. "In the case of Earth's core there is a lot of pressure from the rock in the mantle above which is causing its centre to solidify first. In the absence of a mantle, a core is more likely to start solidifying at the surface. This is exciting because we have never been able to study what this process looks like. Since Psyche cooled so quickly, the mission offers the opportunity to study an entire lifetime of planetary activity" said Bryson.

During the Psyche mission a magnetometer composed of two identical high-sensitivity magnetic field sensors will be attached to the satellite to detect and measure the remnant magnetic field of the asteroid. Other instruments will also measure Psyche's gravity field and elemental composition.

The mission, which was chosen by NASA in January 2017, is targeted to launch in October 2023. It will take six years to travel to the asteroid using solar-electric propulsion, arriving in 2030. Once there, the satellite will orbit the metal world for 12 months, performing its operations from four staging orbits and sending a stream of images and data back to Earth. The satellite will then crash into Psyche, bringing the mission to an end.

[2whatcom-blog]

“Like All Good Stories, It Starts with Pigeons”

On this episode of our podcast My Favourite Theorem, my cohost Kevin Knudson and I have been blissful to speak with Suresh Venkatasubramanian, who's within the laptop science division on the College of Utah. You may hear right here or at kpknudson.com, the place there's additionally a transcript. Dr. Venkatasubramanian likes to explain himself as a bias detective or computational thinker. One of many important focuses of his work previously few years has been on algorithmic bias, the concept that algorithms can reinforce human prejudices. I talked with him for an article I wrote about algorithmic bias for the youngsters's science journal Muse, which can also be obtainable right here, and yow will discover one in all his current papers about algorithmic equity right here. He selected to speak about Fano's inequality, which is vital to his work however has functions rather more broadly in laptop science and statistics. Fano's inequality begins as all good tales do, Dr. Venkatasubramanian says, with pigeons. Particularly, the pigeonhole precept, the intuitively apparent proven fact that if the variety of pigeonholes is smaller than the variety of pigeons you've gotten, a minimum of two pigeons will share one pigeonhole. He describes the way in which the pigeonhole precept varieties the muse for a number of different observations that underlie many decrease bounds in laptop science, together with Fano's inequality. (In laptop science, a typical purpose is to discover a decrease sure for the variety of steps in an algorithm: is there a theoretical minimal period of time it may well take?) Fano's inequality is concerning the quantity of entropy, or uncertainty, in a relationship between two variables. Dr. Venkatasubramanian used the instance of American Caucasian names and genders. Few if any individuals named Nancy are males, and few if any individuals named David are ladies, however there are honest numbers of each women and men (and other people of different genders) named Dylan. So if an algorithm needs to foretell an individual's gender primarily based on their title, it's extra more likely to get it proper if the title is Nancy or David than whether it is Dylan. Fano's inequality makes that, once more intuitively apparent, commentary exact. It places limits on how precisely an algorithm can predict a variable x primarily based on a variable y, primarily based on the uncertainty within the perform that associates these two variables. For extra particulars on Fano's inequality, see Dr. Venkatasubramanian's submit about it right here. A extra superior introduction, by Bin Yu, is right here (pdf). In every episode of the podcast, we ask our visitor to pair their theorem with meals, beverage, artwork, music, or any enjoyment of life. Dr. Venkatasubramanian picked goat cheese and jalapeno jelly. You may should hearken to the episode to search out out why it is the proper accompaniment for Fano's inequality. (For many who wish to see the new pepper-eating orchestra I point out within the episode, the video is right here. However why would you need that?) You will discover Dr. Venkatasubramanian at his web site and on Twitter. He and a few of his colleagues weblog about their work and associated points at Algorithmic Equity. You will discover extra details about the mathematicians and theorems featured on this podcast, together with different pleasant mathematical treats, at kpknudson.com and right here at Roots of Unity. A transcript is obtainable right here. You may subscribe to and overview the podcast on iTunes and different podcast supply techniques. We love to listen to from our listeners, so please drop us a line at myfavoritetheorem@gmail.com. Kevin Knudson's deal with on Twitter is @niveknosdunk, and mine is @evelynjlamb. The present itself additionally has a Twitter feed: @myfavethm and a Fb web page. Be a part of us subsequent time to be taught one other fascinating piece of arithmetic. Beforehand on My Favourite Theorem: Episode 0: Your hosts' favourite theorems Episode 1: Amie Wilkinson's favourite theorem Episode 2: Dave Richeson's favourite theorem Episode 3: Emille Davie Lawrence's favourite theorem Episode 4: Jordan Ellenberg's favourite theorem Episode 5: Dusa McDuff's favourite theorem Episode 6: Eriko Hironaka's favourite theorem Episode 7: Henry Fowler's favourite theorem Episode 8: Justin Curry's favourite theorem Episode 9: Ami Radunskaya's favourite theorem Episode 10: Mohamed Omar's favourite theorem Episode 11: Jeanne Clelland's favourite theorem Episode 12: Candice Worth's favourite theorem Episode 13: Patrick Honner's favourite theorem Episode 14: Laura Taalman's favourite theorem Episode 15: Federico Ardila's favourite theorem Episode 16: Jayadev Athreya's favourite theorem Episode 17: Nalini Joshi's favourite theorem Episode 18: John Urschel's favourite theorem Episode 19: Emily Riehl's favourite theorem Episode 20: Francis Su's favourite theorem Episode 21: Jana Rordiguez Hertz's favourite theorem Episode 22: Ken Ribet's favourite theorem Episode 23: Ingrid Daubechies's favourite theorem Episode 24: Vidit Nanda's favourite theorem Episode 25: Holly Krieger's favourite theorem Episode 26: Erika Camacho's favourite theorem Episode 27: James Tanton's favourite theorem Episode 28: Chawne Kimber's favourite theorem Episode 29: Mike Lawler's favourite theorem Episode 30: Katie Steckles' favourite theorem Episode 31: Yen Duong's favourite theorem Episode 32: Anil Venkatesh's favourite theorem Episode 33: Michele Audin's favourite theorem Episode 34: Skip Garibaldi's favourite theorem Episode 35: Nira Chamberlain's favourite theorem Episode 36: Nikita Nikolaev and Beatriz Navarro Lameda's favourite theorem Episode 37: Cynthia Flores' favourite theorem Episode 38: Robert Ghrist's favourite theorem Episode 39: Fawn Nguyen's favourite theorem Episode 40: Ursula Whitcher's favourite theorem Read the full article

[evitastudio]

Идеально ровный загар мечты.. Без солярия и путешествий. Без побочных эффектов и оранжевых пятен. Нет, Вы не спите! Вы ЗАГОРАЕТЕ ВО СНЕ с несравненным автозагаром James Read! Все что Вам требуется — это нанести средство перед сном, а к утру наслаждаться потрясающим золотистым оттенком кожи! На счету основателя марки Джеймса Рида (James Read) - многочисленные награды бьюти-индустрии, публикации в лучших мировых глянцах и несомненный успех на красных дорожках подиумов. Инновационные продукты James Read произвели революцию в мире домашнего загара: так называемые tan гибриды не просто добавляют фирменное золотистое сияние коже, но и предоставляют комплексный косметологический уход. Эксклюзивная технология Tantone Technology обеспечивает невероятно натуральный загорелый look для всех типов и оттенков кожи. А комплекс активных компонентов, среди которых алоэ вера, экстракты водорослей, гиалуроновая кислота, коэнзим Q10, витамин Е, масло семян жожоба, ухажи��ает за кожей на уровне лучших косметических брендов! Продукты James Read не прилипают к рукам, не оставляют разводов на теле и крайне легко наносятся! Коллекция средств для загара James Read представлена тремя линейками: Self Tan - эффективный природный загар всего за восемь часов. Люксовая косметика в роскошной упаковке с фирменными золотыми полосками и... золотистый оттенок на Вашей коже на долгие дни! Gradual Tan - сияние Вашей кожи круглый год. Контролируйте загар, создавая нужный Вам цвет. Продукты класса «люкс» сочетают уход за кожей с постепенно усиливающимся загаром. Отдельная линейка по уходу за кожей лица. Enhance - раскройте Ваш потенциал загара! С этой линейкой продуктов James Read Ваш загар будет идеальным и на удивление длительным! Мы убеждены: профессиональный загар в домашних условиях по силам каждому. Несравненный загар от James Read ждет Вас в EVITA Studio! Проконсультируйтесь с нашими специалистами для выбора подходящего продукта. Ждём Вас: Evita Studio на Речном + 7 (495) 787 00 57, Evita Studio на Ходынке + 7 (495) 787 00 53

[teded]

How high can you count on your fingers?

How high can you count on your fingers? It seems like a question with an obvious answer. After all, most of us have ten fingers, or to be more precise, eight fingers and two thumbs. This gives us a total of ten digits on our two hands, which we use to count to ten. 

It's no coincidence that the ten symbols we use in our modern numbering system are called digits as well. But that's not the only way to count. In some places, it's customary to go up to twelve on just one hand. How? Well, each finger is divided into three sections, and we have a natural pointer to indicate each one, the thumb. That gives us an easy to way to count to twelve on one hand. 

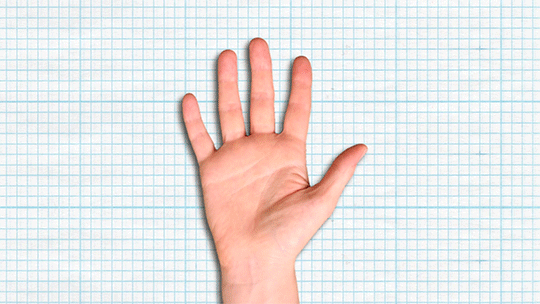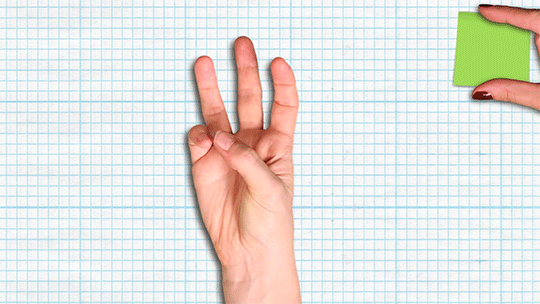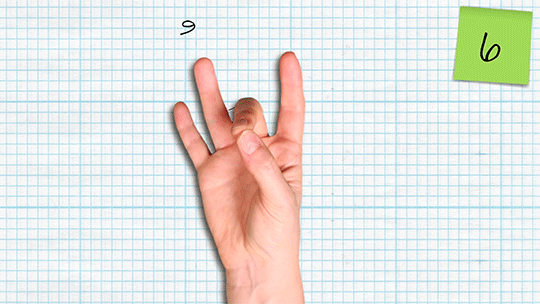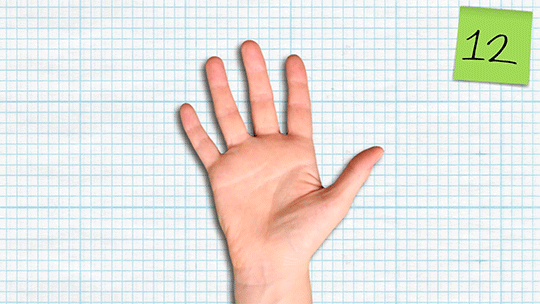
And if we want to count higher, we can use the digits on our other hand to keep track of each time we get to twelve, up to five groups of twelve, or 60.

Better yet, let's use the sections on the second hand to count twelve groups of twelve, up to 144.

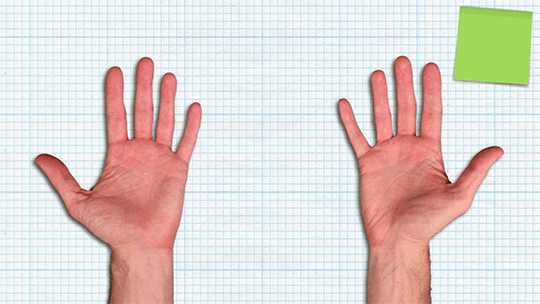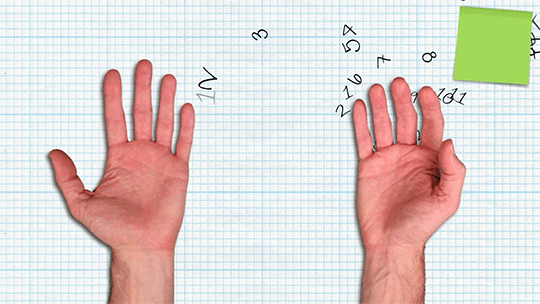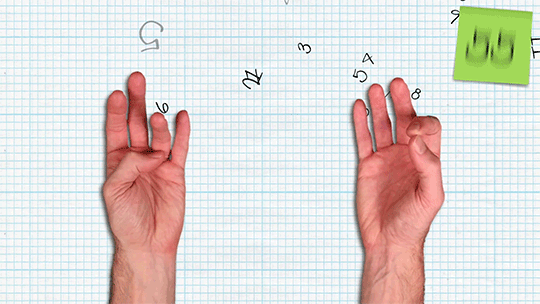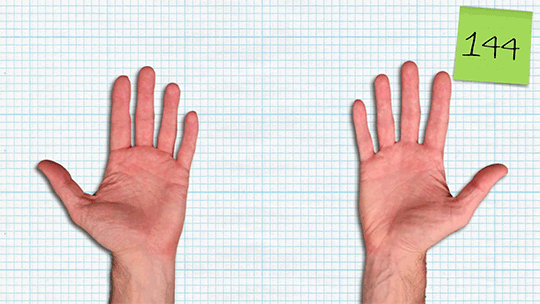
That's a pretty big improvement, but we can go higher by finding more countable parts on each hand. For example, each finger has three sections and three creases for a total of six things to count. Now we're up to 24 on each hand. 

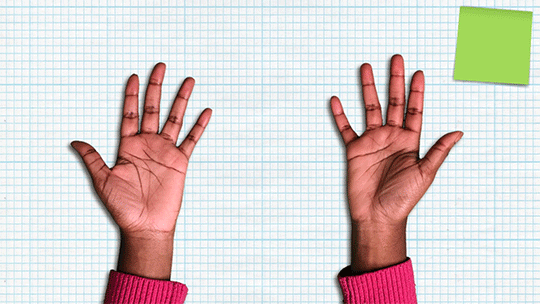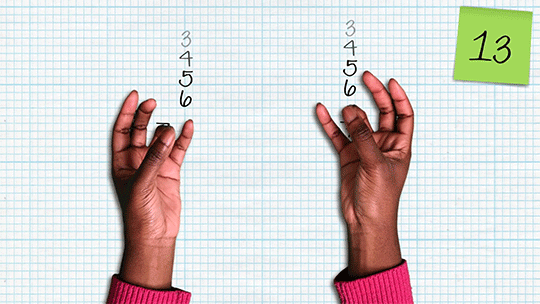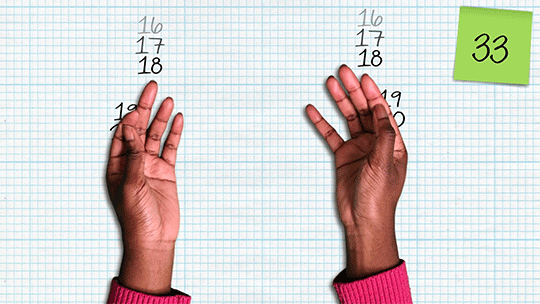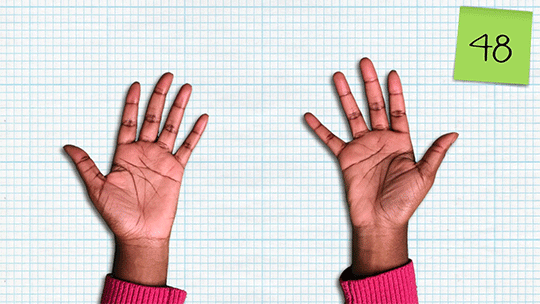
And using our other hand to mark groups of 24 gets us all the way to 576. Can we go any higher? It looks like we've reached the limit of how many different finger parts we can count with any precision. So let's think of something different.

One of our greatest mathematical inventions is the system of positional notation, where the placement of symbols allows for different magnitudes of value, as in the number 999. Even though the same symbol is used three times, each position indicates a different order of magnitude. So we can use positional value on our fingers to beat our previous record. Let's forget about finger sections for a moment and look at the simplest case of having just two options per finger, up and down. This won't allow us to represent powers of ten, but it's perfect for the counting system that uses powers of two, otherwise known as 'binary’. 

In binary, each position has double the value of the previous one, so we can assign our fingers values of 1, 2, 4, 8...all the way up to 512. And any positive integer, up to a certain limit, can be expressed as a sum of these numbers. For example, the number seven is 4+2+1. So we can represent it by having just these three fingers raised. How high an we go now? That would be the number with all ten fingers raised, or 1,023. Is it possible to go even higher? It depends on how dexterous you feel! If you can bend each finger just halfway, that gives us three different states -down, half bent, and raised. Now, we can count using a base-three positional system, up to 59,048. And if you can bend your fingers into four different states or more, you can get even higher. That limit is up to you, and your own flexibility and ingenuity.

Even with our fingers in just two possible states, we're already working pretty efficiently. In fact, our computers are based on the same principle. Each microchip consists of tiny electrical switches that can be either on or off, meaning that base-two is the default way they represent numbers. And just as we can use this system to count past 1,000 using only our fingers, computers can perform billions of operations just by counting off 1's and 0's.

From the TED-Ed Lesson How high can you count on your fingers? (Spoiler: much higher than 10) - James Tanton

Animation by TED-Ed

[noctambulatebooks]

Reading 2017

8-January-2017: Herlihy, James Leo, Midnight Cowboy (1965, USA)

19-January-2017: Zola, Emile, The Conquest of Plassans (1874, France)

22-January-2017: Brooke, Jocelyn, The Scapegoat (1949, England)

24-January-2017: Wharton, Edith, Tales of Men and Ghosts (1910, USA)

10-February-2017: Ainsworth, William Harrison, Old Saint Paul’s (1841, England)

11-February-2017: Vidal, Gore, A Thirsty Evil (1956, USA)

14-February-2017: Perec, Georges, La Boutique Obscure: 124 Dreams (1973, France)

15-February-2017: Modiano, Patrick, Young Once (1981, France)

21-February-2017: De la Roche, Mazo, A Boy in the House (1952, Canada)

27-February-2017: Stapleton, Olaf, Sirius: A Fantasy of Love and Discord (1944, England)

1-March-2017: Modiano, Patrick, La Place De L'Étoile (1968, France)

3-March-2017: Golding, William, Darkness Visible (1979, England)

5-March-2017: Whitman, Walt, The Life and Adventures of Jack Engle (1852, USA)

17-March-2017: Clébert, Jean-Paul, Paris Vagabond (1952, France)

26-March-2017: Gaiman, Neil, The Ocean at the End of the World (2013, England)

1-April-2017: Crowley, John, Beasts (1976, USA)

5-April-2017: Reeves, James A., The Road to Somewhere: An American Memoir (2011, USA)

16-April-2017: Stacton, David, The People of the Book (1965, USA)

26-April-2017: Jünger, Ernst, Storm of Steel (1920, Germany)

20-May-2017: Schumacher, Michael, Dharma Lion: A Biography of Allen Ginsberg (1992, USA)

25-May-2017: Modiano, Patrick, So You Don’t Get Lost in the Neighborhood (2014, France)

29-May-2017: Powers, Richard, Gain (1998, USA)

9-June-2017: Lawrence, D.H., St Mawr (1925, England)

11-June-2017: Kinney, Alison, Hood (Object Lessons) (2016, USA)

15-June-2017: Vidal, Gore, The City and the Pillar (1948, USA)

18-June-2017: Saunders, George, Lincoln in the Bardo (2017, USA)

27-June-2017: Carrington, Leonora, Down Below (1944, England)

2-July-2017: Coover, Robert, The Public Burning (1977, USA)

4-July-2017: Traver, Robert, Anatomy of a Murder (1958, USA)

13-July-2017: Al, Stefan, The Strip: Las Vegas and the Architecture of the American Dream (2017, USA)

16-July-2017: Aramaki, Yoshio, The Sacred Era (1978, Japan)

18-July-2017: Lawrence, D.H., The Man Who Died (1929, England)

23-July-2017: Wharton, Edith, The Mother’s Recompense (1925, USA)

23-July-2017: Wharton, Edith, Madame De Treymes (1907, USA)

27-July-2017: Kipnis, Laura: Unwanted Advances: Sexual Paranoia Comes to Campus (2017, USA)

27-July-2017: Louis, Édouard, The End of Eddy (2014, France)

29-July-2017: Didion, Joan, South and West: From a Notebook (2017, USA)

7-August-2017: Kerouac, Jack, The Sea is My Brother (1943, USA)

12-August-2017: Bishop, John Peale, Act of Darkness (1936, USA)

21-August-2017: Burgess, Anthony, Nothing Like the Sun (1964, England)

28-August-2017: Alexie, Sherman, You Don’t Have to Say You Love Me (2017, USA)

26-September-2017: James, Henry, The Princess Casamassima (1886, USA)

29-September-2017: Kapuściński, Ryszard, The Emperor (1978, Poland)

5-October-2017: Beachy, Stephen, The Whistling Song (1991, USA)

7-October-2017: Grann, David, Killers of the Flower Moon: The Osage Murders and the Birth of the FBI (2017, USA)

21-October-2017: Hardy, Thomas, The Woodlanders (1887, England)

24-October-2017: Simenon, Georges, The Hatter’s Phantoms (1948, France)

25-October-2017: Brainard, Joe, I Remember and Self Portrait (1970-1979, USA)

25-October-2017: Duvert, Tony, Atlantic Island (1979, France)

28-October-2017: Modiano, Patrick, Paris Nocturne (2003, France)

1-November-2017: Germano, William, Eye Chart (Object Lessons) (2017, USA)

7-November-2017: Tanizaki, Junichiro, Devils in Daylight (1918, Japan)

13-November-2017: Pullman, Philip, The Book of Dust (Volume One: La Belle Sauvage) (2017, England)

19-November-2017: Sundstøl, Vidar, The Devil’s Wedding Ring (2015, Norway)

26-November-2017: Ainsworth, William Harrison, Guy Fawkes (1841, England)

12-December-2017: Cohen, Jeffrey Jerome and Linda Elkins-Tanton, Earth (Object Lessons) (2017, USA)

18-December-2017: Dickens, Charles, Great Expectations (1861, England)

21-December-2017: Carrington, Leonora, The Complete Stories (2017, England)

24-December-2017: Babel, Isaac, The Red Cavalry (1926, Russia)

[mathematicianadda]

The Big Lock-Down Math-Off, Match 24

Welcome to the twenty-fourth match in this year’s Big Math-Off. Take a look at the two interesting bits of maths below, and vote for your favourite.

You can still submit pitches, and anyone can enter: instructions are in the announcement post.

Here are today���s two pitches.

James Tanton – Tie folding and a link to Artin’s conjecture

James Tanton is a prolific maths education thinker and the inventor of Exploding Dots. You can find him on Twitter at @JamesTanton where he regularly posts chin-scratching maths problems, or at jamestanton.com.

Who knew that such simple fun as folding a tie can connect with unsolved problems in number theory, and perhaps even provide some new insights?

Clare Wallace – A princess problem

Clare is a mathematician at Durham University. She tweets at @Clare_L_Wallace.

A few months ago, I was volun-told that it was my turn to present at the postgraduate seminar in Probability and Statistics at my university. Never one to miss an opportunity to gain a talk for my own (much less frequent) Pure Maths seminar series, I agreed on the condition of a joint talk between the two groups. Rather than trying to explain my research to two completely different groups of mathematicians, I set out to find some interesting, unintuitive probability questions I could ask my fellow grad students. The Sleeping Beauty Problem is one of them.

Here’s how it works.

Sleeping Beauty has signed up to be part of our psychology experiment. (No princesses were harmed in the making of this pitch!) She’s going to take a sleeping potion on Sunday night, and go and lie down. Then, the researchers will flip a coin. If it’s heads, they will do the Interview Procedure on both Monday and Tuesday; if it’s tails, they will only do it on Monday.

Interview Procedure:

Wake up Sleeping Beauty

Conduct an Interview

Give her two potions to take: a forgetting potion, and a sleeping potion

The forgetting potion means that Sleeping Beauty will not remember whether or not she’s already been interviewed, but she won’t forget the rules of the experiment.

The question is this: Sleeping Beauty is in an Interview, and she’s asked: “From your perspective, what is the probability that the coin landed tails?”

Here it gets tricky.

The argument for 1/2

Heads and tails were equally likely to start with, and Sleeping Beauty hasn’t learned anything: she always knew she would wake up at some point in the process. She has no new information, so she should stick with what she’d have answered before the experiment:

\[ \mathbb{P}(\text{Tails}) = \frac{1}{2} \]

The argument for 1/3

This one is a bit more involved.

Firstly: If the coin landed heads, it’s equally likely, as far as Sleeping Beauty is concerned, that the interview is taking place on Monday or Tuesday.

So

\[ \mathbb{P}(\text{Monday} | \text{Heads}) = \mathbb{P}(\text{Tuesday} | \text{Heads}) \]

We can use a cheeky Bayes’ Theorem here:

\[ \frac{ \mathbb{P}(\text{Monday and Heads})}{\mathbb{P}(\text{Heads})} = \frac{\mathbb{P}(\text{Tuesday and Heads})}{\mathbb{P}(\text{Heads})} \]

On the other hand, learning that it’s Monday doesn’t tell us anything about the coin toss. So

\[ \mathbb{P}(\text{Tails} | \text{Monday}) = \mathbb{P}(\text{Heads} | \text{Monday}) \]

Our old friend Bayes makes a comeback:

[ \frac{ \mathbb{P}(\text{Monday and Tails})}{\mathbb{P}(\text{Monday})} = \frac{\mathbb{P}(\text{Monday and Heads})}{\mathbb{P}(\text{Monday})} ]

And now we’ve convinced ourselves that

\[ \mathbb{P}(\text{Monday and Tails}) = \mathbb{P}(\text{Monday and Heads}) = \mathbb{P}(\text{Tuesday and Heads}) \]

Three equally likely options; nothing else can happen. “Tails and Monday” is the same thing as “Tails”, so

\[ \mathbb{P}(\text{Tails}) = \frac{1}{3} \]

This problem caused a lot of trouble amongst the grad students! By the end of a week of lunchtime discussions, we were (almost) all convinced by the 1/3 argument. My friend Kieran Richards took it one step further, and found a way to switch the probabilities. This time, we roll a six-sided die, and wake up Sleeping Beauty twice if we roll 1, 2, or 3, and once if we roll 4, 5, or 6. In the Interview, Sleeping Beauty is told that the roll was even, and asked “From your perspective, what is the probability that the roll was at least a 4?” In this scenario, following the first argument gives you an answer of 1/3, and following the second gives you an answer of 1/2…

So, which bit of maths made you say “Aha!” the loudest? Vote:

Note: There is a poll embedded within this post, please visit the site to participate in this post's poll.

The poll closes at 9am BST on Saturday the 30th, when the next match starts.

If you’ve been inspired to share your own bit of maths, look at the announcement post for how to send it in. The Big Lockdown Math-Off will keep running until we run out of pitches or we’re allowed outside again, whichever comes first.

from The Aperiodical https://ift.tt/3caKxpK from Blogger https://ift.tt/3ceh7qN

[mrjohnrowe]

RT @jamestanton: Given a square lattice of points it is possible to draw a square that contains in its interior just 1 grid point & a square that contains precisely 4 grid points. It is possible to draw a square that contains precisely 2 grid points. How? [And is there a square that contains 3?] https://t.co/cDk4JytAL8

Given a square lattice of points it is possible to draw a square that contains in its interior just 1 grid point & a square that contains precisely 4 grid points. It is possible to draw a square that contains precisely 2 grid points. How? [And is there a square that contains 3?] pic.twitter.com/cDk4JytAL8

— James Tanton (@jamestanton) December 21, 2019

via Twitter https://twitter.com/MrJohnRowe December 23, 2019 at 12:19AM