#Gottlob Frege

[philosophybits]

The work of science does not consist of creation but of the discovery of true thoughts.

Gottlob Frege, Logical Investigations

[noosphe-re]

One of the great philosophers of mathematics Gottlob Frege made quite an issue of the fact that mathematicians didn't know the meaning of One. What is One? Nobody could answer coherently. Of course Frege answered, but his answer was no better, or even worse, than the previous ones. And so it has continued to this very day, strange and incredible as it is. We know all about so much mathematics, but we don't know what it really is.

Reuben Hersh, What Kind of Thing Is a Number?, A Talk with Reuben Hersh [2.10.97], Edge

[tagitables]

30 October 2024,

I hope to read more about Frege before the year ends.

[geopolicraticus]

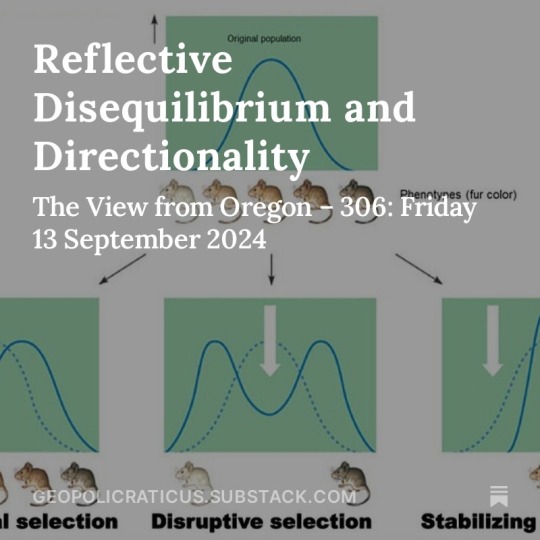
Friday 13 Sept. 2024

Grand Strategy Newsletter

The View from Oregon – 306  

Reflective Disequilibrium and Directionality

…in which I discuss consensus rationality, Galileo, Frege, the Asch conformity experiments, apology tours, equilibrium arbitrage, F. H. Bradley, disequilibrium, directional selection, and Fisherian runaways…

Substack: https://geopolicraticus.substack.com/p/reflective-disequilibrium-and-directionality

Medium: https://jnnielsen.medium.com/reflective-disequilibrium-and-directionality-80bc93960906

Reddit: https://new.reddit.com/r/The_View_from_Oregon/comments/1fhqfuj/reflective_disequilibrium_and_directionality/

[omegaphilosophia]

The Philosophy of Sense and Reference

The philosophy of sense and reference, primarily developed by the German philosopher Gottlob Frege in his 1892 paper "Über Sinn und Bedeutung" ("On Sense and Reference"), deals with the way language and meaning work in relation to objects and concepts in the world. Frege's distinction between sense (Sinn) and reference (Bedeutung) has become a foundational concept in the philosophy of language.

Key Concepts:

Sense (Sinn):

Meaning or Mode of Presentation: The sense of a term or expression refers to the way in which the reference (the object or concept the term refers to) is presented or understood. It is the meaning or the cognitive content associated with the term, which allows us to identify what the term refers to.

Distinct from Reference: Different expressions can have the same reference but different senses. For example, "the morning star" and "the evening star" both refer to the planet Venus, but they have different senses because they describe Venus in different ways.

Reference (Bedeutung):

The Object or Entity Referred To: The reference of a term is the actual object, entity, or concept in the world that the term refers to. It is what the term points to in reality.

Direct Relation to the World: Reference is concerned with the relationship between language and the world, specifically how terms relate to objects or entities that exist independently of our language and thought.

Frege’s Example:

"The Morning Star" vs. "The Evening Star": Frege used the example of "the morning star" and "the evening star" to illustrate his point. Both phrases refer to the same object (Venus), so they have the same reference. However, they convey different meanings or senses because "the morning star" refers to Venus as seen in the morning, while "the evening star" refers to Venus as seen in the evening.

Importance in Philosophy of Language:

Ambiguity and Meaning: The distinction between sense and reference is crucial for understanding how language can sometimes be ambiguous or misleading. For example, different terms might have the same reference but suggest different connotations or associations due to their differing senses.

Identity and Substitution: The distinction also helps explain issues related to identity and substitution in language. For instance, in the sentence "Clark Kent is Superman," the names "Clark Kent" and "Superman" have the same reference (the same person) but different senses (different ways of understanding who that person is).

Impact on Semantics:

Influence on Later Theories: Frege’s work on sense and reference has deeply influenced subsequent developments in semantics, the study of meaning in language. It laid the groundwork for various theories about how language relates to the world and how meaning is constructed.

Distinction from Descriptivism: The sense-reference distinction also contrasts with descriptivist theories of meaning, where the meaning of a name or term is seen as equivalent to a description associated with it.

Applications in Logic and Mathematics:

Formal Systems: Frege’s ideas are also relevant to logic and the philosophy of mathematics, where understanding how symbols and terms relate to objects and concepts is crucial for building formal systems and proving theorems.

Criticisms and Alternatives:

Direct Reference Theories: Some philosophers, like Saul Kripke and Hilary Putnam, have criticized the sense-reference distinction, arguing instead for theories of direct reference, where names and terms refer directly to objects without the mediation of a sense.

Debates in Philosophy of Language: The ongoing debate between Fregean theories and direct reference theories continues to shape discussions in the philosophy of language, especially regarding issues of meaning, reference, and identity.

The philosophy of sense and reference provides a critical framework for understanding how language functions to convey meaning and refer to objects or concepts in the world. By distinguishing between the sense (the mode of presentation) and reference (the actual object), Frege’s theory helps clarify complex issues in semantics, logic, and the philosophy of language, and it remains a foundational concept in these fields.

[h0neyfreak]

kind of insane that Gottlob Frege wanted to eliminate miscommunication with a perfect logical language back in the 1800s and we took his work and used it to build the most perfect miscommunication machine known to man (The Internet)

[perkwunos]

J. Alberto Coffa, The Semantic Tradition from Kant to Carnap

[existentialcomicsfeed]

Frege in the Public Square

[famousdeaths]

Friedrich Ludwig Gottlob Frege was a German philosopher, logician, and mathematician. He was a mathematics professor at the University of Jena, and is understoo...

Link: Gottlob Frege

[peglarpapers]

there comes a time in every university student’s life when they realise that their only hope for getting a relatively okay mark on an essay is to put a knock-knock joke in

[tananansad]

making my language philosophy class notes more digestable as a furry by drawing gottlob frege and bertrand russell as two furries kissing and having steaming hot gay sex

[poniadeaur]

Either the thesis that only what is my idea can be the object of my awareness is false, or all my knowledge and perception is limited to the range of my ideas, to the stage of my consciousness. In this case, I should have only an inner world and I should know nothing of other people -“The Thought: A Logical Inquiry” – Gottlob Frege,

[tagitables]

Gottlob Frege, The Foundations of Arithmetic, A Logico-Mathematical Enquiry Into The Concept of Number

[rhymingslangsblog]

Malbon anomalies

My demons thriving tonight

Tobacco and alcoholic stupor

Regrets in the am

I watch comets and scrawl

Watching the show solo

Red vino

Gottlob Frege turns in his grave

As I declare

I miss you still

xxx

[inthewaytoamultilinguallife]

[Screenshot of black text over a white background that reads: "there are no dubstep albums by Gottlob Frege (the logician who lived in the 1800s); he just did not make any. So the following sentence is not true: (1) There are dubstep albums by Frege"]

thanks

[omegaphilosophia]

The Philosophy of Arithmetic

The philosophy of arithmetic examines the foundational, conceptual, and metaphysical aspects of arithmetic, which is the branch of mathematics concerned with numbers and the basic operations on them, such as addition, subtraction, multiplication, and division. Philosophers of arithmetic explore questions related to the nature of numbers, the existence of mathematical objects, the truth of arithmetic propositions, and how arithmetic relates to human cognition and the physical world.

Key Concepts:

The Nature of Numbers:

Platonism: Platonists argue that numbers exist as abstract, timeless entities in a separate realm of reality. According to this view, when we perform arithmetic, we are discovering truths about this independent mathematical world.

Nominalism: Nominalists deny the existence of abstract entities like numbers, suggesting that arithmetic is a human invention, with numbers serving as names or labels for collections of objects.

Constructivism: Constructivists hold that numbers and arithmetic truths are constructed by the mind or through social and linguistic practices. They emphasize the role of mental or practical activities in the creation of arithmetic systems.

Arithmetic and Logic:

Logicism: Logicism is the view that arithmetic is reducible to pure logic. This was famously defended by philosophers like Gottlob Frege and Bertrand Russell, who attempted to show that all arithmetic truths could be derived from logical principles.

Formalism: In formalism, arithmetic is seen as a formal system, a game with symbols governed by rules. Formalists argue that the truth of arithmetic propositions is based on internal consistency rather than any external reference to numbers or reality.

Intuitionism: Intuitionists, such as L.E.J. Brouwer, argue that arithmetic is based on human intuition and the mental construction of numbers. They reject the notion that arithmetic truths exist independently of the human mind.

Arithmetic Truths:

A Priori Knowledge: Many philosophers, including Immanuel Kant, have argued that arithmetic truths are known a priori, meaning they are knowable through reason alone and do not depend on experience.

Empiricism: Some philosophers, such as John Stuart Mill, have argued that arithmetic is based on empirical observation and abstraction from the physical world. According to this view, arithmetic truths are generalized from our experience with counting physical objects.

Frege's Criticism of Empiricism: Frege rejected the empiricist view, arguing that arithmetic truths are universal and necessary, which cannot be derived from contingent sensory experiences.

The Foundations of Arithmetic:

Frege's Foundations: In his work "The Foundations of Arithmetic," Frege sought to provide a rigorous logical foundation for arithmetic, arguing that numbers are objective and that arithmetic truths are analytic, meaning they are true by definition and based on logical principles.

Russell's Paradox: Bertrand Russell's discovery of a paradox in Frege's system led to questions about the logical consistency of arithmetic and spurred the development of set theory as a new foundation for mathematics.

Arithmetic and Set Theory:

Set-Theoretic Foundations: Modern arithmetic is often grounded in set theory, where numbers are defined as sets. For example, the number 1 can be defined as the set containing the empty set, and the number 2 as the set containing the set of the empty set. This approach raises philosophical questions about whether numbers are truly reducible to sets and what this means for the nature of arithmetic.

Infinity in Arithmetic:

The Infinite: Arithmetic raises questions about the nature of infinity, particularly in the context of number theory. Is infinity a real concept, or is it merely a useful abstraction? The introduction of infinite numbers and the concept of limits in calculus have expanded these questions to new mathematical areas.

Peano Arithmetic: Peano's axioms formalize the arithmetic of natural numbers, raising questions about the nature of induction and the extent to which the system can account for all arithmetic truths, particularly regarding the treatment of infinite sets or sequences.

The Ontology of Arithmetic:

Realism vs. Anti-Realism: Realists believe that numbers and arithmetic truths exist independently of human thought, while anti-realists, such as fictionalists, argue that numbers are useful fictions that help us describe patterns but do not exist independently.

Mathematical Structuralism: Structuralists argue that numbers do not exist as independent objects but only as positions within a structure. For example, the number 2 has no meaning outside of its relation to other numbers (like 1 and 3) within the system of natural numbers.

Cognitive Foundations of Arithmetic:

Psychological Approaches: Some philosophers and cognitive scientists explore how humans develop arithmetic abilities, considering whether arithmetic is innate or learned and how it relates to our cognitive faculties for counting and abstraction.

Embodied Arithmetic: Some theories propose that arithmetic concepts are grounded in physical and bodily experiences, such as counting on fingers or moving objects, challenging the purely abstract view of arithmetic.

Arithmetic in Other Cultures:

Cultural Variability: Different cultures have developed distinct systems of arithmetic, which raises philosophical questions about the universality of arithmetic truths. Is arithmetic a universal language, or are there culturally specific ways of understanding and manipulating numbers?

Historical and Philosophical Insights:

Aristotle and Number as Quantity: Aristotle considered numbers as abstract quantities and explored their relationship to other categories of being. His ideas laid the groundwork for later philosophical reflections on the nature of number and arithmetic.

Leibniz and Binary Arithmetic: Leibniz's work on binary arithmetic (the foundation of modern computing) reflected his belief that arithmetic is deeply tied to logic and that numerical operations can represent fundamental truths about reality.

Kant's Synthetic A Priori: Immanuel Kant argued that arithmetic propositions, such as "7 + 5 = 12," are synthetic a priori, meaning that they are both informative about the world and knowable through reason alone. This idea contrasts with the empiricist view that arithmetic is derived from experience.

Frege and the Logicization of Arithmetic: Frege’s attempt to reduce arithmetic to logic in his Grundgesetze der Arithmetik (Basic Laws of Arithmetic) was a foundational project for 20th-century philosophy of mathematics. Although his project was undermined by Russell’s paradox, it set the stage for later developments in the philosophy of mathematics, including set theory and formal systems.

The philosophy of arithmetic engages with fundamental questions about the nature of numbers, the existence of arithmetic truths, and the relationship between arithmetic and logic. It explores different perspectives on how we understand and apply arithmetic, whether it is an invention of the human mind, a discovery of abstract realities, or a formal system of rules. Through the works of philosophers like Frege, Kant, and Leibniz, arithmetic has become a rich field of philosophical inquiry, raising profound questions about the foundations of mathematics, knowledge, and cognition.