#Cognitive Arithmetic

[omegaphilosophia]

The Philosophy of Arithmetic

The philosophy of arithmetic examines the foundational, conceptual, and metaphysical aspects of arithmetic, which is the branch of mathematics concerned with numbers and the basic operations on them, such as addition, subtraction, multiplication, and division. Philosophers of arithmetic explore questions related to the nature of numbers, the existence of mathematical objects, the truth of arithmetic propositions, and how arithmetic relates to human cognition and the physical world.

Key Concepts:

The Nature of Numbers:

Platonism: Platonists argue that numbers exist as abstract, timeless entities in a separate realm of reality. According to this view, when we perform arithmetic, we are discovering truths about this independent mathematical world.

Nominalism: Nominalists deny the existence of abstract entities like numbers, suggesting that arithmetic is a human invention, with numbers serving as names or labels for collections of objects.

Constructivism: Constructivists hold that numbers and arithmetic truths are constructed by the mind or through social and linguistic practices. They emphasize the role of mental or practical activities in the creation of arithmetic systems.

Arithmetic and Logic:

Logicism: Logicism is the view that arithmetic is reducible to pure logic. This was famously defended by philosophers like Gottlob Frege and Bertrand Russell, who attempted to show that all arithmetic truths could be derived from logical principles.

Formalism: In formalism, arithmetic is seen as a formal system, a game with symbols governed by rules. Formalists argue that the truth of arithmetic propositions is based on internal consistency rather than any external reference to numbers or reality.

Intuitionism: Intuitionists, such as L.E.J. Brouwer, argue that arithmetic is based on human intuition and the mental construction of numbers. They reject the notion that arithmetic truths exist independently of the human mind.

Arithmetic Truths:

A Priori Knowledge: Many philosophers, including Immanuel Kant, have argued that arithmetic truths are known a priori, meaning they are knowable through reason alone and do not depend on experience.

Empiricism: Some philosophers, such as John Stuart Mill, have argued that arithmetic is based on empirical observation and abstraction from the physical world. According to this view, arithmetic truths are generalized from our experience with counting physical objects.

Frege's Criticism of Empiricism: Frege rejected the empiricist view, arguing that arithmetic truths are universal and necessary, which cannot be derived from contingent sensory experiences.

The Foundations of Arithmetic:

Frege's Foundations: In his work "The Foundations of Arithmetic," Frege sought to provide a rigorous logical foundation for arithmetic, arguing that numbers are objective and that arithmetic truths are analytic, meaning they are true by definition and based on logical principles.

Russell's Paradox: Bertrand Russell's discovery of a paradox in Frege's system led to questions about the logical consistency of arithmetic and spurred the development of set theory as a new foundation for mathematics.

Arithmetic and Set Theory:

Set-Theoretic Foundations: Modern arithmetic is often grounded in set theory, where numbers are defined as sets. For example, the number 1 can be defined as the set containing the empty set, and the number 2 as the set containing the set of the empty set. This approach raises philosophical questions about whether numbers are truly reducible to sets and what this means for the nature of arithmetic.

Infinity in Arithmetic:

The Infinite: Arithmetic raises questions about the nature of infinity, particularly in the context of number theory. Is infinity a real concept, or is it merely a useful abstraction? The introduction of infinite numbers and the concept of limits in calculus have expanded these questions to new mathematical areas.

Peano Arithmetic: Peano's axioms formalize the arithmetic of natural numbers, raising questions about the nature of induction and the extent to which the system can account for all arithmetic truths, particularly regarding the treatment of infinite sets or sequences.

The Ontology of Arithmetic:

Realism vs. Anti-Realism: Realists believe that numbers and arithmetic truths exist independently of human thought, while anti-realists, such as fictionalists, argue that numbers are useful fictions that help us describe patterns but do not exist independently.

Mathematical Structuralism: Structuralists argue that numbers do not exist as independent objects but only as positions within a structure. For example, the number 2 has no meaning outside of its relation to other numbers (like 1 and 3) within the system of natural numbers.

Cognitive Foundations of Arithmetic:

Psychological Approaches: Some philosophers and cognitive scientists explore how humans develop arithmetic abilities, considering whether arithmetic is innate or learned and how it relates to our cognitive faculties for counting and abstraction.

Embodied Arithmetic: Some theories propose that arithmetic concepts are grounded in physical and bodily experiences, such as counting on fingers or moving objects, challenging the purely abstract view of arithmetic.

Arithmetic in Other Cultures:

Cultural Variability: Different cultures have developed distinct systems of arithmetic, which raises philosophical questions about the universality of arithmetic truths. Is arithmetic a universal language, or are there culturally specific ways of understanding and manipulating numbers?

Historical and Philosophical Insights:

Aristotle and Number as Quantity: Aristotle considered numbers as abstract quantities and explored their relationship to other categories of being. His ideas laid the groundwork for later philosophical reflections on the nature of number and arithmetic.

Leibniz and Binary Arithmetic: Leibniz's work on binary arithmetic (the foundation of modern computing) reflected his belief that arithmetic is deeply tied to logic and that numerical operations can represent fundamental truths about reality.

Kant's Synthetic A Priori: Immanuel Kant argued that arithmetic propositions, such as "7 + 5 = 12," are synthetic a priori, meaning that they are both informative about the world and knowable through reason alone. This idea contrasts with the empiricist view that arithmetic is derived from experience.

Frege and the Logicization of Arithmetic: Frege’s attempt to reduce arithmetic to logic in his Grundgesetze der Arithmetik (Basic Laws of Arithmetic) was a foundational project for 20th-century philosophy of mathematics. Although his project was undermined by Russell’s paradox, it set the stage for later developments in the philosophy of mathematics, including set theory and formal systems.

The philosophy of arithmetic engages with fundamental questions about the nature of numbers, the existence of arithmetic truths, and the relationship between arithmetic and logic. It explores different perspectives on how we understand and apply arithmetic, whether it is an invention of the human mind, a discovery of abstract realities, or a formal system of rules. Through the works of philosophers like Frege, Kant, and Leibniz, arithmetic has become a rich field of philosophical inquiry, raising profound questions about the foundations of mathematics, knowledge, and cognition.

[teledyn]

The Psychological Scaffolding of Arithmetic

"We used to think that if we knew one, we knew two, because one and one are two. We are finding that we must learn a great deal more about ‘and’."

— Sir Arthur Eddington

https://psycnet.apa.org/fulltext/2023-84614-001.html

[mateooo88]

I was thinking about the psychological profile, philosophical profile, symbolism, and overall analysis of fyodor, and thought I'd share it :3 (also requested by my friend, zizi!!!)

Fyodor is definitely not insane. He is clarity distilled into cruelty, his intelligence is sharp enough to wound, and his presence can be seen as quiet, mannered, even tender at times, feels less like calm and more like still water above a black trench. He is the kind of man who could kill you mid prayer and whisper 'May God forgive us both.'

If you were to look at him from a clinical perspective, Fyodor presents as a high functioning psychopath, he lacks empathy yet understands it well enough to counterfeit it when needed. He has no interest in domination through brute force. Instead, he opts for control through inevitability—turning the enemy’s own mind, morals, and fears against them.

3 major parts of the psychological side of him can be,

• Cognitive empathy — He understands how people think, what they fear, what they value

• Moral detachment – He does not recognize the sanctity of life— only the sanctity of order or ideology.

• Narcissistic asceticism — His self-denial (minimalist dress, avoidance of indulgence) is not humility, but superiority disguised as piety, he is above worldly pleasures because he seeks something beyond the world.

moving onto the philosophical side of him,

Fyodor embodies the nietzschean ubermensch warped into spiritual nihilism, where dostoyevsky the author once wrote, in the brothers karamozov, "If God does not exist, everything is permitted" In the show, fyodor reverses the lens, “Because God does exist, everything must be cleansed.”

He isn’t an atheist, he’s worse. He’s a theological fatalist. To him, suffering is divine currency. Death is not evil; it is purifying. The world is a broken equation and Fyodor believes he is the godsent variable to correct it, even if that means breaking every soul to do it. (which you can label as a savior complex.)

He sees morality as a human construct, fragile and flawed. Instead, he moves according to cosmic arithmetic; cold logic, divine punishment, and a sense of destiny that puts him outside the reach of conventional good or evil. To him, "Mercy is weakness. Pain is clarity. Death is math."

and then, the symbolism,

In mythology, rats are both survivors and symbols of plague. Fyodor aligns with this duality; he spreads rot, but always escapes the trap.

His black clothing can be seen as a reflection of mourning, but not his own. He is eternally dressed for the funeral of mankind.

The name, itself, “Dostoyevsky”, A callback to themes of guilt, divine justice, and inner torment, but unlike the original Dostoyevsky’s characters, BSD Fyodor has killed his conscience and called it enlightenment.

Lastly, id like to talk about his relationship to death and power,

Fyodor doesn’t fear death, instead he romanticizes it. He treats life like a riddle, unsolvable while inside it, perfectly logical in the rearview. To him, people are merely pieces, and he doesn't move them with hatred or pleasure. He moves them like a mathematician balances an equation. Efficiently. Without waste.

He isn't in love with power in the traditional sense. He desires finality, the collapse of corrupt systems, the silencing of chaos, the 'correct' end of the human narrative. If others must die to reach that still point, so be it. In fact, so must he. His own life is just a tool for his design.

Overall, Fyodor Dostoyevsky is the kind of man who could burn down a city and call it mercy. He is not chaos for chaos’s sake—but order so absolute that it becomes indistinguishable from annihilation.

He is the devil in the mirror of God.

A poet of quiet ruin,

and his verses are written in blood, logic, and silence.

[chthonic-cassandra]

If the dislocation in the structure of creation [as described by Marx] were, as in the Judeo-Christian scriptures, described in narrative rather than embedded in two disparate cognitive styles (the sensuous rendering of the interior of capital and the arithmetic rendering of the embodied worker), if it were rendered imagistically rather than analytically, the action of the story might be summarized in this way. In the midst of a vast industrial plain stood an artifact, a commodity, a pile of luminous coal so glittering with reflected sunlight that it seemed to belong to a world of heat, yet so deep and dark in its purple and blue that its blackness seemed not just its color but the very thing that it once must have been, something far removed from the sunlit surface of the plain. Two men crossed the plain, approached the commodity, and stood on either side of it. The one extended his arm and touched the artifact and, as he did so, his body grew larger and more vivid until all attention to his personhood or personality or spirit was made impossible by the compelling vibrancy of his knees, back, hands, neck, belly, lungs: even the interior of his body stood revealed in small cuts and larger wounds. Simultaneously, the other extended his arm and touched the artifact and as he did so, his body began to evaporate, grow airy: he was spiritualized, and disappeared. A name was given to each of the two: in his bodily magnification, the first was called by the name "worker"; in his bodily evaporation, the second was called by the name "capitalist." The two belonged to two tribes who, though they inhabited the same Palin (where one produced coal and the other was warmed by it), never confronted one another face to face, for though the location of the first was apparent to the second, the location of the second was unknown to the first: having no body, he was grounded in no specific location; his face was hidden, invisible; or rather, he did not have a face if by face is meant bodily tissue subject to frostbite. Scattered across this part of the plain were many such artifacts (some were piles of coal, others bolts of cloth, others books, others beds) framed by many such pairs of men. Elsewhere, not far away, on a different part of the same plain stood a pile of money. Like the coal pile, the money pile was an artifact, though far less sensuous in its appearance than the first (for on this plain of the relation between persons and artifacts, it is not just men and women but artifacts themselves that display varying degrees of de-sensualizaton and dematerialization). It too was framed by men, five on one side and one on the other. As at the earlier site, all extended their arms and touched the artifact and, as they did, the five on one side became larger and more intense in their physical presence, while the one on the other side was sustained in his disappearance. Still further along on this same vast plain was a small pile of paper-halfway-transformed-into-an-idea. Although this third artifact was less compelling in its sensuous appearance than the second, as the second was less sensuous than the first, its powers to regulate the appearance and disappearance of persons was correspondingly greater than the second, as the powers of the second were greater than those of the first. Framing this pile of paper were fifty men on one side and one on the other, and once more the arms of all were extended: the fifty persons became fifty enlarged bodies and the one disappeared from the plain and could not be found, though his voice was present, even omnipresent, in the social rules, legislation, and philosophic assumptions that swirled across the plain like an angry wind that was felt on the embodied faces of those who remained.

Elaine Scarry, The Body in Pain: The Making and Unmaking of the World

[gil-estel]

gonna force everyone I know to read this article

Abstract

High-quality mathematics education not only improves life outcomes for individuals but also drives innovation and progress across society. But what exactly constitutes high-quality mathematics education? In this article, we contribute to this discussion by focusing on arithmetic fluency.

The debate over how best to teach arithmetic has been long and fierce. Should we emphasize memorization techniques such as flashcards and timed drills or promote “thinking strategies” via play and authentic problem solving? Too often, recommendations for a “balanced” approach lack the depth and specificity needed to effectively guide educators or inform public understanding. Here, we draw on developmental cognitive science, particularly Sfard’s process–object duality and Karmiloff-Smith’s implicit–explicit knowledge continuum, to present memorization and thinking strategies not as opposing methods but as complementary forces. This framework enables us to offer specific recommendations for fostering arithmetic fluency based on the science of learning. We define arithmetic fluency, provide evidence on its importance, describe the cognitive structures and processes supporting it, and share evidence-based guidance for promoting it. Our recommendations include progress monitoring for early numeracy, providing explicit instruction to teach important strategies and concepts, implementing well-structured retrieval practice, introducing time-limited practice only after students demonstrate accuracy, and allocating sufficient time for discussion and cognitive reflection. By blending theory, evidence, and practical advice, we equip educators and policymakers with the knowledge needed to ensure all children have access to the opportunities needed to achieve arithmetic fluency.

[maths-screaming]

Reading Books on Dyscalculia

Books on dyscalculia may have started this process, but they haven't been terribly helpful mid-journey.

Which isn't to say I don't recommend them! Especially if you are a parent or teacher of students with dyscalculia, or if you are trying to understand your own dyscalculia!

It's just that I expected them to offer more insight into the condition and more concrete ways to address it, and most of them...aren't. At least, they're not telling me anything I haven't figured out on my own from re-learning basic arithmetic with current best practices in teaching.

The facts are:

We don't actually know all that much about dyscalculia currently. The reams of research on dyslexia don't have a counterpart for dyscalculia. That research is currently being done - it's a very exciting time to be in the field - but it'll be 10-20 years before you and I can just go read works on dyscalculia that have the same depth and rigor as works on dyslexia do now.

We know even less about dyscalculia in adults. Less of everything. We know less about how it affects adult lives, how it affects adult cognition, how adults work around it, and how to teach adults.

Good luck finding resources on dyscalculia in teens and adults tackling advanced math classes. The overwhelming majority of resources are for teaching early number sense, counting, and basic arithmetic.

On point 3, I think of myself as an ad hoc test subject: I'm going to find out if I learn* algebra, trig, and stats more easily once I finally understand basic arithmetic. I'm going to see if the strategies and approaches I'm using for relearning basic arithmetic carry through, and if so, how far. I haven't yet found any books or resources that answer that question for me.

But if you are a parent or teacher of kids with dyscalculia and/or you want to read up on what we do know, here's what's on my reading list currently:

Dyscalculia: Action Plans for Successful Learning in Mathematics, by Glynis Hannell. An overview of teaching/tutoring strategies for early learners. Most of these recommendations will work best in a one-on-one or small group setting, so I'd actually recommend this book for parents more than for teachers.

The Dyscalculia Resource Book: Games and Puzzles for Ages 7 to 14, by Ronit Bird. A collection of games and puzzles to teach number sense, arithmetic facts families, and so on. Pretty thin on the why and how behind dyscalculia, though.

The Trouble With Maths: A Practical Guide to Helping Learners With Numeracy Difficulties, Steve Chinn. Steve Chinn's name appears on several books on dyscalculia at this point (not all of which I could find through interlibrary loan). This book is a blend of theory and practice; it's not exactly recreational reading, but it's very insightful.

---

*I'd say "re-learn," but I didn't really learn any of middle or high school math - at least not in a way that allowed me to retain any of it, with the notable exception of geometry.

[ahuactl]

on mathematical models

the question “is math invented or discovered?” remains to be the most contentious questions in mathematics.

a significant portion of people including mathematicians believe that mathematics holds objective truth insofar as their models, theorems and equations tell the truth about reality in which the Subject: their proposition exists independently of them. this position is familiar among those in the Platonist or Realist traditions of mathematics. common knowledge states that any proposition such as  P: 1 + 1  = 2  is true in which ever universe it is stated in such that its truth is not constructed upon the confines of our society and more strongly, not in our minds or cognitions.

i will present my argument here on why i disagree with this view. first, propositions like P: 1 + 1  = 2 are certainly not true in every construction of a mathematical system. for example, in boolean algebra and nonstandard number systems. even non-Platonist mathematicians would quickly disagree with this as they would claim that the Platonist view holds that the truth of a proposition exists not solely but with the pair (P, S) whereas P is the proposition and S is the system in which it is considered to be in. on such note, this leads me to my next argument.

from the development of set theory in the 19th century, it was discovered by mathematicians that mathematics as a field was built on shaky logical foundations (re: Russel’s Paradox). this thus necessitated the construction of a new structure which must possess the power to rebuild mathematics. this new foundation came with the introduction of ZFC. it is an attempt to systematically rebuild the previously known and accepted results of mathematics while working to eliminate the logical inconsistencies of previous naive set theory. from how it was made, ZFC does not posit the truth of any propositions insofar that it exists as starting axioms in which rules of inference can be applied. as with any theories of logic, its axioms must be decided and chosen. it could be noted that these axioms were posteriori in a sense that they were built to solve the issues such as Russel’s Paradox. it can then be argued that the process by in which these axioms were chosen is contingent upon the values of the persons that chose it as well as the developed culture of mathematics. in fact, it is by no means that ZFC is only the possible theory of mathematics (e.g. Model Theory, Category Theory). no matter the “objective” metaphysical existence of mathematical objects, by the way mathematics was built and practiced, these starting axioms still remain a choice.

to reiterate, the way mathematics has been agreed to be used by mathematicians, whichever system set in place still remains a choice. this reveals its use as pragmatic: the system, that is, ZFC was adapted because it works. this naturally extends out from its foundations to concrete mathematical objects studied in abstract algebra, analysis, etc. for example, a group is not just a set with specific rules with its binary operation; the construction of the properties that denote a Group came a posteriori in a way that it was discovered that the set of properties in the first place proved to be a useful abstraction to study. 

on a related note, i would argue that the existence of abstract mathematical objects actualize themselves by our need and desire for the world to conform to standard rules as appeal to our intuition and senses. the truth value of P: 1 + 1  = 2 in (P, S) in an arithmetic system S signifies an essence in which it “makes sense” that combining abstract objects  result to a set in which it succeeds singular objects by the value of each one of those objects. the value of (P, S) does not reveal a Platonic truth of our universe but instead a declaration of common sensical deductions. in such, a mathematical statement is a “model” the same way a bachelor is “unmarried”.

on usage:

regardless of the metaphysical existence of mathematical objects, it, as a system, is undoubtedly one of the finest works of humankind. i advise that one ought not to use this powerful tool to make claims of absolute truth. in a sense, the existence of mathematical objects remain irrelevant to how it ought be used. one should not at all invoke mathematics to justify repressive systems and to cause grave harm to humanity. as a tool, it must not be used for evil. one must always remain cautious of individuals who use mathematics as arguments by certainty. these individuals who swear by the sharp sword of mathematics is most likely using it to silence thought and keep one from questioning. always look at the mathematics; an equation is not just a set of symbols that denote some property; it is a theory of the world and this theory must be met with most criticisms when it is being used to make claims for human policies. look at the mathematics, specifically which assumptions were made by the ones who present them: it must reveal the true character of such persons.

[justinspoliticalcorner]

Greg Owen at LGBTQ Nation:

In video shared by Fox News on Monday, Donald Trump again claimed schools around the country are “taking” kids and performing “operations” on them. “There are some places your boy leaves the school, comes back a girl,” Trump insisted without proof. “Okay? Without parental consent. What is that all about? That’s like — when they talk about a threat to democracy, they’re a threat,” Trump continued, apparently referring to his Democratic opponent and clearly rattled that her message about his authoritarian nature and cognitive decline are taking hold.

In a rambling answer to a Bronx dad at what the New York Post described as a “surprise” visit to a barbershop packed with Black men who support Trump, the former president, slumped in a barber chair, held forth incoherently on his solution for problems at America’s schools: stopping teachers and administrators from transitioning kids. All of this was in response to the dad’s question about how Trump would help Bronx public schools perform better – the dad didn’t mention trans kids at all. “Could you imagine without parental consent? At first, what I was told that was actually happening, I said, you know, it’s an exaggeration. No. It happens! It happens in areas where it happens. We’re not going to let it happen, but we are going to straighten out a lot,” Trump said, untethered from the facts or syntax.

[...] “I never saw – you don’t need any of them. You know, I want one person and a secretary to just make sure they’re teaching English. Okay. Give a little English,” he said. “Okay? I say reading, writing, and arithmetic. No transgender, no operations.”

Donald Trump pushing anti-trans nonsense yet again, this time by falsely asserting that public schools are helping kids transition and perform gender-confirmation surgery operations.

See Also:

The Advocate: Donald Trump, an actual threat to democracy, claims it’s transgender kids who are a ‘threat to democracy’

[isthenapoleoncute]

This Napoleon is doing some hard thinking!

A lot of people don’t know this, but Napoleons have some remarkable problem-solving and cognitive abilities. They have been found to have an advanced level of cognitive power, above that of even a four-year-old human child. They can understand cause and effect, and use tools to solve problems, such as using a stick to obtain food from a hard-to-reach place, or using artillery to decimate infantry to make way for cavalry. They can even comprehend and use arithmetic, counting well above the number eight.

Studies have found that Napoleons have proportionaly similar sized brains as many primates, which is thought to be the reason for their advanced intelligence.

But their intelligence doesn't stop there, Napoleons have been found to be able to understand, use and combine concepts such as same and different, big and small, and different colors and shapes. They are able to understand the concept of zero and use it to solve problems, demonstrating a level of cognitive sophistication.

Pretty cute, if you ask me!

[hannah-snow]

Girl Math. Girl Driving. Ladybrain.

Ugh. Those things drive me insane.

I hope it isn't the trad community making those jokes, because who's better at math than a trad girl? Who's a better driver than a mother who has to navigate the school drop-off in a car? Who's juggling more stuff on less sleep than anyone else? Women, that's who.

What does cooking and baking require? Rations, proportions, fractions, quantity judging, and spatial awareness!

What does money management require? Arithmetic!

What does knitting, crochet, and sewing require? G E O M E T R Y baby, it's a working exercise in geometry!

What does motherhood involve? High levels of (executive) function, common sense, emotional intelligence, and cognitive and affective empathy.

What does running a household involve? Management skills!

Don't ever let anyone tell you that women are dumb, ladies. Our smarts and ingenuity are just as important to running this world as mens are.

And if I ever get to be a tradwife, I don't just want to sit there mincing around with my latte. I want to take satisfaction in a difficult and worthwhile career well done. Like my current teaching career.

[anaxerneas]

If we remain with Husserl, we have to say that mental processes are one with their phenomenality.

To think a real number — say, 2 — or to perform a basic arithmetical operation with regards to it (say 2 + 3 = 5) is utterly to exhaust its phenomenality. There is nothing other than cognition at issue. Yet when I perceive, remember, anticipate or even imagine two pears, their phenomenality falls outside the immanence of consciousness, such that I am in principle required to embark on an infinite task of description, even though I may have very high levels of Evidenz at my disposal with respect to the pears if I can perceive them with more than one sense. The number 2, as I apprehend it in consciousness, does not need to be reduced; it does not give itself to me in any orientation with regard to consciousness at all. Yet the two pears make endless demands upon me because I can see them from different perspectives.

If Husserl tells us, time and again, about this, it is nonetheless to the painters, photographers, sculptors and poets that we must go for concrete testimony. Often when literature is at issue we are directed (and, first of all, by Henri Maldiney) to Francis Ponge, to elaborate texts such as "Le Verre d'Eau" or "Le Savon," and with good reason. Here, though, I would like to cite Wallace Stevens' poem, "Study of Two Pears"...

Kevin Hart, "The Irreducible"

[psychdorian]

How Insomnia Affects Kids

Insomnia is normally studied in adults, because of the severe side effects on the body and a person’s ; one big side-effect can be on memory.  In adults, sleep is important to declarative memory because it helps with memory formation.  It can cause even bigger issues if the insomnia disorder is continued (i.e. chronic insomnia) [1].  BUT: For kids declarative memory is ALSO heavily impacted by sleep.  In a study on declarative memory (a) in children and adults, both groups were better at remembering things after sleeping that after trying to remember things after staying awake [2]. (A nap, a goodnights sleep etc. was all helpful for remembering).

Studies like this are interesting in a time where sleep has become so diminished in kids and even adults, because the effects on memory are actually pretty large.  

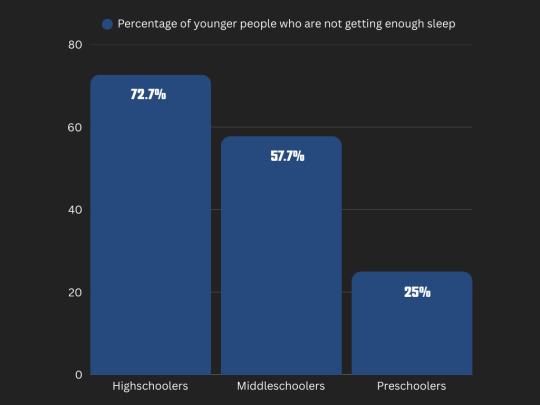
On weekdays ~72.7% of highschoolers sleep less than the recommended 8 hours a night for this age group [3]   

57.8% of middle schoolers sleep less than the recommended 9-12 hours of sleep a night for this age group [4]. 

Considering the amount of sleep kids are getting and how many of them have insomnia, this might be even more worrying.  

In childhood ~30% of children have insomnia [5]; in adolescence 20-25% have insomnia [3].  

What’s really crazy is that even younger than adolescence, even younger than childhood: 

~25% of preschoolers have sleep issues, and ~16.6% have insomnia [6]. 

Kids at all ages can have insomnia and serious sleep issues. They are something to pay attention to, they will affect kids in all kinds of way.

GOOD NEWS: If you’re interested in learning about insomnia’s effects on declarative memory (or memory in general) right now there is a lot more research available on the topic than there was about 10 years ago.  Especially concerning children/teenagers etc. there’s been more of a development on looking at this topic, and ways to help improve sleep for younger people specifically. Some Interesting Treatments at the moment: 

Pharmaceutical Treatment (drugs/sleep meds): benzodiazepine and even non-benzodiazepine medications are pretty popular.  They’re mainly for anxiety/insomnia, and normally used for adults (because these may cause issues with dependency, and potential abuse of medication, they are a last resort for children and adolescence). 

Therapy: Cognitive Behavioral Therapy (CBT) – This is a kind of talk therapy, normally it’s pretty helpful if the insomnia is comorbid with something else like anxiety.

The current problem with treatment is that: insomnia treatment varies wildly from person to person; often once treatment ends of either pharmacological treatment or CBT, patients will relapse [7].

Hopefully research on the causes of insomnia in kids continues as well as research on treatments.  Because of the impact on learning and memory specifically, it’s very worrying the amount of kids with insomnia and sleep issues, so many parents have been looking at options for treatment lately as people are more comfortable relying on psychiatric help.  Insomnia is a big deal, it has a lot of negative effects, the more interest people show in getting help for it, the better the research will get. …

(a) Declarative Memory: Memory of facts and information– so for children/teenagers this could be, arithmetic, history, English, foreign language lessons, etc. [1] [2] [3] [4] [5] [6] [7]

[daisybslt]

Speech & Language Pathology Stroke Terms

Disorganised list of terms used in slt for stroke- for study/quick reference.

AAC- Alternative augmentative communication.

ABI- Acquired brain injury (anytime after birth).

Agnosia- Inability to process sensory information (in Greek gnosis- "not knowing").

Aphasia- Impairment of language, affecting the production or comprehension of speech and the ability to read or write, depending on the affected area of the brain. (Greek a/without + phásis/speech").

Apraxia- Inability to execute purposeful, previously learned motor tasks, despite physical ability and willingness. (Greek a/without + praxis/action).

Aspiration- Food or fluid accidentally enters the lungs through the windpipe, often as a result of dysphagia.

Bilateral- Involving or affecting both sides, usually referencing both sides of the body.

Capacity- Ability to reason, make decisions and consider choices, express views and receive and understand information (AWI= Adult With Incapacity).

Cerebro-Vascular Accident (CVA)- Stroke.

Cognitive function- Anything involving thinking.

Deep Vein Thrombosis (DVT)- Blood clot forms in a deep vein.

Deglutition- The act of swallowing.

Dementia- Decline of function in the brain causes cognitive problems such as difficulty with memory, understanding and mood.

Dysfluency- Any type of speech which is marked with repetitions, prolongations and hesitations; an interruption in the flow of speech sounds. Commonly known as stuttering or stammering.

Dysarthria- Collection of motor speech disorders.

Dysphagia- Swallowing difficulties.

Embolism- Blockage of a blood vessel by a blood clot or piece of fatty material or other debris in the blood stream. An embolism is a type of stroke.

Enteral feeding- Feeding through a tube connected to the person's stomach.

FAST test- 'Facial drooping, Arm weakness, Speech problems, Time to call 999'

Fluency- Smoothness with which sounds, syllables, words and phrases are joined together during oral language.

Hemorrhagic stroke-  Caused when a blood vessel in the brain bleeds into the brain tissues or the space around the brain.

Hemianopia-  Loss of one half of your visual field. 

Hemiparesis- Weakness on one side of the body.

Hemiplegia- Complete paralysis on one side of the body.

Hypotonia- Abnormal decrease of muscle tone.

Ischemic stroke/ Infarct- Damage to the brain caused by lack of blood flow, usually from a clot. An area of tissue that is dead because of a loss of blood supply. This is the most common type of stroke.

Infarction- A sudden loss of a tissue’s blood supply causing the tissue to die.

Ischemia- Blood flow (and thus oxygen) is restricted or reduced in a part of the body. 

Ischemic penumbra- Areas of damaged but still living brain cells arranged in a patchwork pattern around areas of dead brain cells.

Lacunar stroke/infarct- When a small artery deep in the brain becomes blocked, causing a small area of damaged brain tissue.

Left hemisphere- Controls speech, comprehension, arithmetic, and writing.

Muscle tone- The degree of natural tension in a person's muscles. Abnormally high muscle tone can lead to muscle tightness and stiffness (spasticity). Very low muscle tone can cause floppiness (hypotonia).

Muscle tension- When muscles of the body remain semi-contracted for a period of time in the resting state.

Naso-gastric (NG) tube-  Medical catheter that's inserted through your nose into your stomach.  Used both to deliver substances to your stomach and to draw substances out.

Neglect- Not being aware of one side of the body and/or environment.

Neuron- Nerve cell.

Neuroplasticity- The ability of undamaged parts of the brain to take over the jobs of damaged areas.

Nystagmus- Continuous uncontrolled movement of the eyes.

Paralysis- Partly or entirely unable to move the affected parts of the body.

Percutaneous endoscopic gastrostomy (PEG)- Feeding tube placed through the abdominal wall and into the stomach.

Right hemisphere- Controls creativity, spatial ability, artistic, and musical skills

Stroke- Occurs when something blocks blood supply to part of the brain or when a blood vessel in the brain bursts.

Spasticity- Abnormal increase in muscle tone or stiffness of muscle

Thrombolysis- An early treatment for some types of strokes caused by a blood clot.

Thrombosis- A blood clot that forms in an artery.

Transient Ischaemic Attack (TIA)-  sometimes called a “mini-stroke”. Blood flow to the brain is blocked for a short time, usually no more than 5 minutes.

Vertebral artery dissection (VAD)- A tear in the walls of an artery at the back of the neck, blood can then get between the layers of artery walls and can lead to a clot forming, causing a blockage (a stroke).

Visual field loss- The loss of sight in a particular area of visual field.

[bruinsmontessori]

Unlocking Learning Potential with Authentic Montessori Tools in the USA

When a child’s eyes light up after solving a hands-on math problem, it’s more than just academic success—it’s the Montessori method in action. Designed to empower children with independence and confidence, Montessori education uses thoughtfully crafted materials to build cognitive, sensory, and motor skills. Among these, Montessori bead material USA and Montessori cylinder block USA are two of the most iconic tools that help children internalize abstract concepts through tangible experiences. This article delves into the importance of these materials, how they support developmental milestones, and why authenticity matters when choosing educational tools.

THE ROLE OF MONTESSORI MATERIALS IN EARLY EDUCATION

Montessori materials are rooted in the idea that children learn best through manipulation and exploration. Tools like the Montessori bead material USA are specifically designed to represent mathematical concepts such as units, tens, hundreds, and thousands in a visual and tactile way. These hands-on materials are more than just educational toys—they serve as bridges between concrete understanding and abstract thinking. When children handle bead chains and golden beads, they gain a real sense of quantity, pattern, and sequence. This builds not only math proficiency but also confidence and autonomy in learning.

WHY MONTESSORI BEAD MATERIAL MATTERS

The Montessori bead material USA is a staple in many classrooms and homes because it supports sequential learning in a multisensory format. Children use color-coded beads to understand number progression, place value, and arithmetic operations. For example, the transition from single digits to the decimal system becomes clear when children can physically count and compare bead bars. Using these materials, students learn at their own pace, reinforcing lessons through repetition and practice. Quality and precision in these tools are essential, as they must align perfectly with Montessori pedagogy to be effective in fostering meaningful learning experiences.

THE PHILOSOPHY BEHIND MONTESSORI TOOLS

Montessori education emphasizes self-directed activity, hands-on learning, and collaborative play. Each material has a specific purpose and is introduced in a carefully planned sequence. Rather than memorizing facts, children are encouraged to explore and discover. This method allows learners to develop critical thinking and problem-solving skills naturally. Materials like the bead chains and number tiles provide children with immediate feedback, making learning more intuitive. The structured freedom offered by Montessori materials empowers children to develop focus, discipline, and a lifelong love for learning.

UNDERSTANDING THE MONTESSORI CYLINDER BLOCK

Equally vital to Montessori classrooms is the Montessori cylinder block USA, which supports the development of visual discrimination and fine motor coordination. Children engage with cylinder blocks by removing and replacing different-sized cylinders into corresponding sockets, honing their grasp of dimension and depth. Midway through the learning process, the repetitive practice of these activities strengthens both concentration and spatial awareness. Using the Montessori cylinder block USA, children begin to grasp foundational concepts that later support geometry, measurement, and analytical thinking. This material lays the groundwork for more complex learning by training the senses in a structured and engaging way.

ADVANTAGES OF USING QUALITY CYLINDER BLOCKS IN THE USA

The Montessori cylinder block USA is more than a sensory tool—it’s a foundational piece that supports a child’s journey toward logical reasoning and problem-solving. High-quality versions of this material are crafted to precise specifications, allowing children to explore differences in height, width, and depth with accuracy. These blocks are designed to be durable and safe, ensuring they meet the standards of both home and classroom environments. Authentic Montessori materials are essential for preserving the integrity of the method, especially when it comes to delivering consistent educational results. Children thrive when given tools that align with developmental principles.

CHOOSING THE RIGHT SUPPLIER FOR AUTHENTIC MATERIALS

Selecting authentic Montessori tools is crucial for educators and parents who wish to provide a rich, purposeful learning environment. It is important to consider not just the design but also the material quality, safety, and adherence to Montessori standards. Inconsistent or poorly crafted tools can hinder learning and create confusion, especially in early developmental stages. That’s why turning to reputable sources is key. One such provider known for reliable and high-quality Montessori materials is bruinsmontessori.com, offering trusted options for those looking to enrich their children’s education with certified tools that meet the method’s true intent.

CONCLUSION: THE POWER OF MONTESSORI MATERIALS IN SHAPING FUTURES

Montessori education continues to impact learners by giving them the tools to understand the world through exploration and discovery. With materials like the Montessori bead material USA and the Montessori cylinder block USA, children are not just memorizing—they're experiencing learning in a meaningful way. The value of authentic materials lies in their ability to connect theoretical ideas to physical understanding. For those seeking dependable and high-quality options, bruinsmontessori.com offers a range of materials that support genuine Montessori learning. Investing in the right tools today can make a lasting difference in a child's educational journey.

[so-true-overdue]

The Gospel of Immunity: A Sermon on the Sacred Shield of Vaccination

Behold, the paradox of human cognition: we champion progress, yet tremble before its fruits.

In a world spinning upon the axis of relentless biological warfare—our cells besieged by microbial marauders—vaccines stand as sentinels, the silent paladins of immunological defense. Yet, their divine efficacy is often questioned by those who worship at the altar of anecdote, forsaking the empirical cathedral of science.

Consider the Magnitude of Their Grace

Vaccines are not mere concoctions of modern alchemy. They are the crystallized wisdom of centuries, born of Pasteur’s tenacity, Jenner’s courage, and the accumulated trials of countless minds wielding microscopes like swords. They have subdued polio’s paralysis, exorcised smallpox’s specter, and shielded countless millions from the venoms of measles, tetanus, and pertussis.

And what of their adversaries? The infinitesimal specter of adverse reactions. Let us quantify the peril: the risk of a severe allergic reaction to a vaccine—a dreaded anaphylaxis—is approximately one in a million. To contextualize, the chance of being struck by lightning in one’s lifetime is 1 in 15,300. Shall we eschew umbrellas next? Or perhaps condemn electricity itself as too great a hazard to endure?

On Negotiation with Risk

Vaccination is not the obliteration of risk; it is a calculated negotiation with uncertainty. To vaccinate is to weigh the scales of harm and benefit, to face the specter of a one-in-a-million reaction and declare that the lives of millions are worth that gamble. Contrast this with the toll exacted by diseases unopposed: measles alone claims the lives of over 100,000 annually, most of them children. This is not conjecture—it is arithmetic, immutable and unyielding.

Shall we refuse the shield because it may chafe? Shall we spurn the ship because it cannot promise calm seas? Such is the folly of those who elevate potential discomfort above the preservation of collective health. They barter statistical insignificance for certainty of peril, trading herd immunity for herd vulnerability.

The Poetry of Immune Memory

The true marvel of vaccination is not merely its prevention but its pedagogy. Vaccines instruct our immune system, training it to recognize the invader before the battle has begun. They are rehearsals for wars that may never come. To vaccinate is to inscribe within the body a symphony of defense, a living testament to humanity’s ingenuity and resilience.

Without this foresight, the immune system becomes a tactician unprepared for the ambush. Disease ravages unchecked, leaving scars upon both flesh and society. Vaccines, therefore, are not just medicinal; they are ethical. They embody the moral imperative to protect not only oneself but the vulnerable—the infant, the immunocompromised, the elder.

A Closing Admonition

Let us not falter in the face of misinformation, for it spreads with a virulence rivaling the diseases we seek to defeat. Let us arm ourselves with knowledge and statistics, with reason and compassion. The act of vaccination is an act of faith—not blind faith, but faith rooted in evidence, nurtured by science, and borne aloft by the unassailable truth that humanity is stronger united.

Go forth, then, and spread the gospel of immunity. For in the armory of progress, the needle is mightier than the microbe.

[t-allyitup]

ed edd n eddy alphabet soup part ii anyone?

may be headed in the direction of a fic me thinks idk i'm just at a point where my brain is experiencing a creative outpouring in the form of ed edd n eddy content

[eddeddy specific i guess. let's see]

cw for substances, implied (but up to interpretation) traumatic events, child abuse/domestic violence mention, double d enjoying calculus

[also ... i may have mentioned this previously somewhere but i'm currently almost 2 years clean from dxm abuse & all drugs so PLEASE know that interactions with substances are not something that should be romanticized or promoted, i'm not trying to do that i just also have been in high school and as a teenager substances are something that exist]

[for purposes of me being me this story features the three ed's as seniors in high school, as well as rolf, kevin, and nazz. johnny & may kanker are juniors in high school, sarah & jimmy are sophomores, and eldest kankers have graduated hs]

[eddy's brother is in the can and staying there cuz i'm not about to deal with his ass sorry]

picking up from double d's brain alphabet soup...

- beginning

but that wasn't productive ruminating for the current task at hand, no.

no, the focus of his current endeavor, as he had been sorely forcing his near non-existent attention span back too, was familiarizing himself with the revision outline for the first calculus ii quiz of his last year of high school.

calculus had been relatively simple, the theoretical aspect of geometry soothingly absent from this branch of arithmetic, with many of the classic formula/solve/answer questions (however tedious the problems may be.) double d enjoyed problems with direct, proven answers, especially ones that would simply be labeled as correct or incorrect. knowing that without a doubt there was a proven, logical, factual answer for a problem was motivating and provided structure.

although he still received excellent markings in his literature classes, and had become especially gifted in theoretical analysis and research investigation, the concept of language left him feeling rather uncomfortable. while there were specific outlines, literary devices, and general frame of composition to guide him, a compelling and well-written piece of writing was largely subjective. personal opinions of the audience were essential to the response of the piece, and therefore effective criticism stemmed often from personal preference rather than execution.

ed, a third of double d’s nearly life-long trio of best friends, had surprisingly excelled in the areas of creative writing and storytelling in the past few years. while his spelling and grammar needed (and likely would always need) some work, his ability to reveal emotion in his writing was pleasantly shocking and impressive to both edd and eddy. double d had scanned ed’s last few impromptu creative writing assignments, and had beamed with pride at each one, offering only some minor suggestions each time that the other teen had taken with great appreciation and warmth. ed had also grown to become interested in cooking, and after nearly burning double d’s house to ash multiple times while trying to cook hot dog burgers, had gotten a strict warning that he'd better work on his scientific skills if he ever considered returning to double d's kitchen again. this had led to double d spending a few grueling hours explaining measurements and proper handling of equipment, but had ultimately paid off in the form of the trio enjoying a delicious ed-cooked meal nearly three times a week.

ed had also joined a d&d league, following a recommendation from a (long overdo) psychiatric assessment that ed had dyslexia and adhd, and would benefit from some time outside of his house. double d and eddy had understood that this was a nice way of the shrink telling ed's parents that their continued domestic violence mishaps and borderline abusive behavior towards their son had been resulting in cognitive decline, and that ed having a productive hobby and solid support system would hopefully be able to keep him stable and shield him from his parents further traumatizing him.

eddy was, to few of the cul-de-sac kid's surprise, quite gifted in the areas of communications, business, and physical education. following his brother’s arrest and eventual incarceration, eddy had been hitting the gym to try and kill off his festering anger and hurt from the abuse. he had made the junior varsity football team the first year, and was quickly promoted to varsity his sophomore year. eddy was still shorter than ed and double d, but was noticeably stronger and better ‘filled out.’ eddy had prided himself on his self-described 'total beefy hunk of a bod,' much to ed's howls of amusement and double d's eye roll of affection. while eddy worked tirelessly on his car, ranting about the 'idiotic lack of, if actually existent, formation strategy’ of the quarterback, double d had become increasingly aware of how eddy's personality had developed. it was clear that eddy had become more confident, in not only himself, but in his abilities. he also showed an impressive talent for pattern recognition, strategizing, and planning. this had been a major asset to their football team, who were mostly 6'2 boulders that had clearly only made the team for their size. of course, it was inevitable that eddy would always be the snarky little attention hog he was, but it was pretty clear to double d that eddy was also becoming observant and (though this may be a stretch) stabilized. he had also gotten tattoos, one of a dragon that matched with ed and a few for other reasons that double d hadn't been paying attention to due to his uncharacteristic staring in shock and (now identifiable) gay panic. eddy had developed an obsession with piercings, too. his ears, left eyebrow, right nostril, and tongue in specific were victims of this endevor, and although kevin had rolled his eyes and scoffed at the look, eddy looked incredible and had remained confident in his appearance.

in fact, it was jimmy, who had grown up more than anyone could imagine, who was the first to compliment eddy on his piercings. jimmy had taken up swimming and diving, as well as student government, and debate team with eddy. his voice was scratchy and soothing, and his ability to tear it up in the pool had astonished and overjoyed everyone. he had been returning to states for swim year after year since the 7th grade, and the legendary smile that had always been the focal feature of his face was now accompanied by a freckling of acne. his baja sweatshirts and acid wash jeans, along with a surprising choice of reebok shoes, had been proof of his own self-settling.

sarah had become distant, although physically present, halfway through freshman year. that october, a rumor had circulated about sarah that double d didn't even want to think about, especially after sarah had told him the truth about what had actually happened.

sarah stayed close to ed the next few years, the rare instance of a unmistakable clang of a sarah laugh present after a particularly goofy joke from jimmy or a swat at the hand of ed trying to steal a french fry was rare, but had become more common. the sound of her laugh was helpful for reminding them that she was healing, and that the blazing force of fire that lies inside, although flickering, hasn't gone out.

johnny had joined a d&d leauge with ed, and was unsurprisingly terrific at arts of all kinds. he had gotten lead roles in the last few student-led visual arts productions, twice as the main supporting character and once as the lead-joseph, in 'joseph and the amazing technicolor dreamcoat.' in the summers, johnny had played baseball, and was playing varsity by junior year. plank remained lodged in his backpack, a comforting reminder that some things never change.

rolf had also become interested in musical theater, as well as baseball. during baseball season, rolf, johnny, and kevin would spend lots of time together, baseball having brought them back. kevin and rolf remained close year round, rolf supporting kevin during football season and kevin supporting rolf at his theater performances, although theater was ‘nerdy’ in his unwarranted opinion.

to everyone's immense surprise, nazz had joined a band. she had joined a rock-n-roll jazz band with marie kanker and rolf, as well as two other boys and the occasional sarah on backup vocals. nazz had also joined the d&d league, per request of ed, and had seemed to be enjoying that. in the summer she played softball, also with marie, and had spent a great portion of each season trying to convince sarah to play.

nazz had grown into someone that double d deeply admired. she was authorative, inclusive, open, and stood firm in herself and her beliefs. she hadn't been interested in showing off, focusing primarily on connection and including others. the other boys in his grade had teased her for her interest in make up and fashion, and nazz didn't care. she enjoyed ‘feminine’ things because they made her happy, just like shredding on her guitar and playing d&d and other typically ‘masculine’ things made her happy. she did what she wanted to do, and she had told double d that even if she was applying make up or learning how to kick start a car engine to impress someone, than what's so wrong with that? what's wrong with wanting to look nice for someone, or wanting to be able to help them? what's wrong with having pride in yourself?

double d had went home after that conversation and started crying. he couldn't stop, and he didn't understand why he had been crying in the first place. he wasn't much of a crier at all-in fact he hadn't cried in months-but when nazz had explained that to him, he supposed he realized how much he cared about her. he realized that the kind of growth it takes to reach that conclusion could only stem from something changing, and he realized that an inevitable and painful part of growth is change.

he had also realized that change triggered advancement, which triggered the discovery of philosophies like the one nazz lived by.

it had also come to mind that to develop such a welcoming and independent philosophy, nazz may have experienced something that was possibly quite traumatizing.

it was the same string of thoughts that double d had regarding the look on eddy’s face before they parted ways for the night. the humid july night buzzed with cicadas and secondhand smoke hung suspended under the artificial buzz of the street light near their houses. a roar of laughter from eddy followed by a the scheming, sly smirk, the kind where you try to stop your teeth from showing but it happens anyway, the light producing a halo effect upon his best friend and leaving double d in a glowy haze. the way eddy’s nose scrunches slightly with the smile and the way his deep, spruce blue eyes stare coyly at him before he takes a final drag of a blunt rolled in a way the should be considered a violation of the herb's personal rights. his eyes mischievous but distant as his lips and lungs kill the joint for good, the image of eddy flicking the discarded paper away and exhaling the smoke upwards. following this dramatic gesture, was what eddy had said to double d moments before he had said goodnight on that warm july evening.

'd, there's no point in me stayin’ after graduation. nothin’ left to stay for once you and lumpy leave for school. i'm leaving this shithole, one way or another.’

double d remembers that moment exactly. the moment that triggered everything to follow, the specific moment when he had realized that he was absolutely terrified of losing his best friend in a way that hadn't previously aligned with his stasis levels. he remembers eddy waving goodbye, calling something about ‘catching him later,’ and double d paced back home and locked himself in his room.

he thought of what nazz had said earlier that day when the older kids of rhe cul-de-sac had gathered at rolf's for table tennis and an excuse to finish a pack of twisted teas. that it isn't wrong to want. it was, in fact, a fundamental function of a sentient creatures existence, and that the external shame that people project on others for having desires in inherently hypocritical. nazz, with her laid back attitude and approachable demeanor, sharing the wisdom of a life once lived through self-criticism.

he thought of what eddy had said about leaving this place, one way or another, and it was then that double d let the pieces of his strained conscious unravel and descended into a fit of choking through tears.

it was becoming very obvious to that eddward that he was entering an entirely different plane of trouble.

- end

if i spelled something wrong no i didn't . gn