#gödels theorems

[noosphe-re]

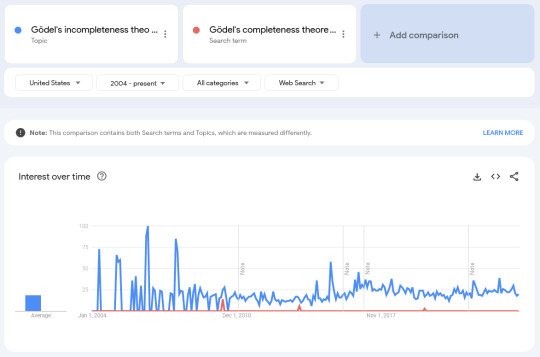
In 1931, the Austrian logician Kurt Gödel pulled off arguably one of the most stunning intellectual achievements in history. Mathematicians of the era sought a solid foundation for mathematics: a set of basic mathematical facts, or axioms, that was both consistent — never leading to contradictions — and complete, serving as the building blocks of all mathematical truths. But Gödel’s shocking incompleteness theorems, published when he was just 25, crushed that dream. He proved that any set of axioms you could posit as a possible foundation for math will inevitably be incomplete; there will always be true facts about numbers that cannot be proved by those axioms. He also showed that no candidate set of axioms can ever prove its own consistency. His incompleteness theorems meant there can be no mathematical theory of everything, no unification of what’s provable and what’s true. What mathematicians can prove depends on their starting assumptions, not on any fundamental ground truth from which all answers spring.

Natalie Wolchover, How Gödel’s Proof Works, Quanta Magazine, July 14, 2020

[maxwellshimbo]

RIP Kurt Gödel you would have loved "What if kinkshaming was my kink?"

[rippledawn]

Had a fun time this afternoon

Was hanging out with some of my friends who are also mathematicians, and as mathematicians do we of course started spontaneously talking about deep philosophical topics like what is information, and does mathematics exist independently from intelligent life to interpret it

Could not find a nerdier conversation topic, but we had a fucking blast

God I love being a mathematician

[girlonthelasttrain]

where's that xkcd comic about overestimating how much the general public knows about any specific scientific subject. gotta make a version for mathematics and logic...

[chevril-glove]

I love this so much

When I was a kid, I remember being really astounded by the fact that arithmetic is consistent. Like uh, 2 + 3 + 4 is 9. And 9 - 4 is 5, which is 2 + 3, and 9 - 2 is 7, which is 3 + 4! And 7 is also 6 + 1, and guess what, 9 is also 6 + 1 + 2, and even more amazingly, 6 is 4 + 2, and (remember 9 - 4 is 5) 2 + 2 + 1 is 5! No matter what you do it's always consistent, you can't trick it by adding and subtracting some convoluted sequence of numbers until it gives you an answer that's inconsistent with the rest.

When I was first learning arithmetic I remember that I just found this crazy, like, how does it always work out? That shouldn't be possible. And I figured that, naturally, when I got older, someone would eventually give me the answer to this incredibly pressing question. And, well, it turns out nobody has the answer, and they actually proved that it's impossible for anyone to give a satisfying answer. So, that's a bit disappointing.

[i-iii-iii-vii]

[auporias]

the amount of space that gödels incompleteness theorem takes up in my head is unreal

like the idea that any sufficiently complex consistent formal system cannot be complete (there are always truths inaccessible from within a formal system)

so sometimes we have to step outside of the rigidities of structure in order to access truth

if this interests you, the book gödel escher bach by douglas hofstadter is an incredible trip

[arachnion]

GQ: Why do you like math more than Korean?

WT: Yes. I think that if you do Korean somehow, you can find the answer. But numbers, math, are so certain. So, if I'm wrong, I can openly admit it and say, 'I was wrong.' That's really great. (Laughs)

WINTER x GQ KOREA 2024 WOMAN OF THE YEAR

[lifesfeelings]

Incompleteness Theorem

Okay, so that whole last post got y’all ready for another lesson on something that I find interesting and want to just write about 1) to remember the information but 2) teach others about it/ask real knowledgeable people to teach me! So, tldr on my last post, I have entered a math fixation in my learning, though, not how to do it but rather the logics and theories behind it. With that, I just started reading “Gödel, Escher, Bach.” I’m only like 50 pages in and I did hear someone online say it took him 7 years to read it lol!! but it’s FASCINATING so far! And don’t quote me on being any kind of knowledgeable person when it comes to this: I don’t do math and I study English. But I just wanted to take some notes on what I’ve learned! So this actually goes way back to a Veritasium video, “Math’s Fundamental Flaw,” which taught on Gödel’s “Incompleteness Theory.” In terms of how Hofstadter tells it: no system is able to be “completed” in that there are no set of proofs that can both 1) prove all statements in a system to be universally true and 2) not self-contradict itself at some point. I guess at its core that basically there will always be truths in math that are true but not provable. The proofs are simply too weak to ever actually reach certain truths without then creating paradoxes in the system. Hofstadter actually starts the book first though by describing Bach’s music and Escher’s art as these things called “strange loops” in which they both will seemingly move forward in a direction (Bach’s music would go up in pitch or Escher’s drawing would show stairs going upward), and yet always return to the it’s original place—the music would return to its original key and the stairs would return to the bottom of the painting. I’m honestly so scared for the rest of this book just because the complexity it’s described with on Wikipedia. I don’t know if half of it will make sense and apparently there’s all these hidden themes and patterns in the Achilles stories he tells too? I’m not good at picking out hidden shit like that and I feel like half of it will go over my head haha! If I got anything wrong or if people want to just sdd to this post I would LOVE that :)

[its-a-hil]

*holds out my hand* we can fix this. together

[in-sightpublishing]

Conversation with Tomáš Perna on AI and ANN: Member, World Genius Directory (4)

                      Publisher: In-Sight Publishing Publisher Founding: March 1, 2014 Web Domain: http://www.in-sightpublishing.com Location: Fort Langley, Township of Langley, British Columbia, Canada Journal: In-Sight: Independent Interview-Based Journal Journal Founding: August 2, 2012 Frequency: Three (3) Times Per Year Review Status: Non-Peer-Reviewed Access: Electronic/Digital & Open…

[4denthusiast]

I've just noticed an odd combination of beliefs I hold about how maths works. I'm not exactly convinced that every statement in arithmetic (i.e. statements that can be written in terms of 0, the successor function, =, +, ×, and, not, and quantification over natural numbers) is either true or false. (My previously more Platonist views were shaken by some university courses on topics like model theory and Gödel's incompleteness theorems. In order to understand that stuff you have to entertain the possibility that certain seemingly obvious things aren't true, and I then sort of never stopped for some of them.) At the same time, I accept the law of the excluded middle (that P∨¬P is always true). I generally wouldn't describe myself as an intuitinist, even if I am interested in the applications of some intuitionistic logics in computer science.

I think the way I resolve this apparent contradiction is that the reason I don't feel like all arithmetic statements are true or false is that I'm not sure the natural numbers, as a set, are uniquely defined. Any definition of what is and what isn't in ℕ tends to involve some degree of circularity. "It's 0, and S0, and SS0, and so on.", but "so on" for how many steps? A natural number of steps. Hopefully you see the issue. So then, an arithmetic statement may be true of one model of the naturals, but not another. Within any one model, P∨¬P is true (or, more to the point, (∀x. Px)∨¬(∀x. Px) is true), so if it's true in every model it's true, but we can't ever pin down quite which model we're talking about, so the individual statement (∀x. Px) can remain indeterminate.

All this sort of implicitly relies on a separation of the language and meta-language, even though I didn't set out to have a separate meta-language in the first place. I'm not quite sure whether what I'm thinking here even makes sense. Perhaps what I mean is that the meta-language does have logical connectives (and, or, not), so you can form a claim like "(∀x. Px) is true, or ¬(∀x. Px) is true.", but it doesn't have quantification over the naturals, at least not always, because in the meta-language there isn't a unique ℕ, and you can't specify which one you mean because there's no way to totally pin it down. At least I think. But then the semantics of A∨B is meant to be that A∨B is true iff A is true or B is true. I guess we can still recover this by saying that any statement in the language that includes any quantifiers is implicitly with reference to a particular model of ℕ, and a statement is true iff it's true for all models, but then that requires that the meta-language can quantify over models of ℕ, which should be way less possible than quantifying over individual naturals. I don't know how to resolve this, if it even can be resolved. I'm kind of confused.

The true ℕ, if it exists, ought to be the smallest one of course. The trouble is you can't define "smallest" properly without discussing the whole class, which is a less basic concept than the numbers themselves. Also, not every ordered set or class has a smallest element. I think probably if you allow yourself sufficient expressiveness you can prove that in this case there is a smallest (take the intersection or something), but again I don't think you can prove that without making assumptions at least as strong as the conclusion.

The same thing happens with set theory, but there it all feels clearer. In contrast to the naturals where I'm not sure, I feel somewhat more confident that there isn't a single true set-theoretic universe V. There ought to be sets that can't be named (there are only countably many names after all), which makes the universe much trickier to pin down than the naturals. I know there are countable models of ZFC, but they don't feel like they're the real model, and ZFC is itself kind of vague. It leaves a lot of room for rather natural variation in what sets are allowed (e.g. the continuum hypothesis), while non-standard naturals seem much more exotic. If you assume that there's some particular ℕ that's the real ℕ, in the meta-language, this gives you much more solid foundation to use when talking about potential uncertainty in V. You can do induction, talk about constructible sets and stuff. It seems quite likely that the continuum hypothesis doesn't have a definite truth value, even though CH∨¬CH does, but it feels like quite an ordinary sort of indefiniteness, like "He has brown hair." when it isn't clear who "he" refers to. "Is there a cardinal between ω and 2^ω?" What version of the class of cardinals are you talking about?

[max1461]

I *think* the deal with Gödel's incompleteness theorems is that it's just a cardinality thing, it's like a pigeonhole principle thing (in spirit; it's not literally the pigeonhole principle). Like that's sort of what diagonalization arguments are, and Gödel has a diagonalization argument. If you set up some system of rules, there will always be consequences of that system that you can't prove because you're limited to "too few possible proofs". I think even Gödel himself said something to this effect somewhere but I can't find it.

It's like. Imagine some sentence in PA that's like ∀nP(n), right. Well it might be unprovable in PA. In fact, Con(PA) is of exactly this form: "∀n(n does not encode a valid derivation of a contradiction)" or whatever, and it's unprovable in PA. But from the outside ∀nP(n) is still true in the sense that, well, no matter how many numbers n you check, you'll never find one that doesn't satisfy P. In fact, any statement of the form ∀nP(n) which is unprovable must be true, because if it were false we could find a counterexample. But we can't make that inference from within PA, we have to make it "on the outside".

I find this sort of unsettling because...

Nevermind. I don't find it unsettling any more. I think it's normal and no big deal. There are definitely interesting issues around this but I think in itself it's kind of no big deal. Especially if you're like, a Curry style formalist and you think of math as the empirical study of the behavior of systems of rules and proofs as repeatable experiments (reducing math to metamath, and making it a science).

It's just all, all Gödel is saying is that mathematical systems are "too small" to directly tell you everything about themselves. Again, *I think*. It's like, no more mysterious than the halting problem, because it literally is the halting problem. I don't think the halting problem is that wacky either. Ok there's no algorithm that tells you whether other algorithms will halt. Sure, an algorithm for a decision problem is a pretty restricted sort of thing. It's not really a surprise that there isn't one that does that.

Anyway the upshot is I don't think Gödel forces us to be any more Platonist than we already are. Like in general, even without Gödel, if you want to be a game formalist, say... and you claim "I've proven φ from the axioms of PA", and you want that to be interpreted in roughly the same way as "I made the move ke4 in a chess game", or whatever... well, to interpret the latter claim, I have to ask questions like "what's a chess game?", "what does it mean to make a move?", and these still get me into the territory of ontology of abstract objects and semantics of claims about abstract objects. So game formalism doesn't save you from this sort of thing. And conversely I don't think Gödel produces any more issues of this kind. If I claim "∀nP(n) is true but unprovable in PA", the thing is it might be hard for me to know I'm right (because ∀nP(n) is uprovable, so I have to investigate empirically, à la Curry's idea), but if the trouble is over the semantics, well, I'm still just making a truth claim about an abstract object (the system PA), so it's like. We're semantically in no more dubious territory than with the claim about making a move in chess or the claim about a totally non-Gödelian proposition proved in PA. It's just that it's harder to establish our claim as true.

[yesthatsatumbler]

I'm reminded of the Letters from the Future project.

OK, so you probably wouldn't have heard about it. TL/DR: in 2002, there was a website where people could send messages to... um... let's say a girl from the future (...also from some kind of weird AU where her adventures happened in the 2170s and not the 2070s as the usual descriptions would have it), and get answers from her, for a while. The project functioned in this manner only for a few months; then the interaction part closed down (there were some vaguely-related fanworks posted on the same site later) but the existing letters remained up (I just checked and they're still up as I write this).

I found the site as an impressionable teenager in 2007, very much liked it, and for a while legitimately believed that the letters must have been arriving from some kind of future. Until I found the Fermat's Last Theorem comment.

Slightly paraphrasing: she said that Fermat's Last Theorem was still unsolved in her time, and that there was a strong suspicion that it was actually unprovable [in the Gödelian sense], but the attempts to prove that hadn't succeeded yet either.

Now, I could plausibly assume that an author in 2002 would have been unaware of Wiles, and/or, semi-equivalently, that by the 2180s some kind of error would have been found in his proof. (I think I knew about the DS9 joke/retcon by then; if not, I'd have read about it shortly afterwards.)

The part that made absolutely no sense to me in an "actual letters from a smart future girl" context was the suggestion that 1) FLT could have been unprovable; and, more importantly, 2) that this in turn is something that could be proved.

You can't prove that FLT is unprovable. It's absolutely, mathematically impossible. And this is still true even if you don't accept (or know about) the Wiles proof; it's a pretty simple argument that only requires relatively simple math.

...OK, now I should probably do the actual math.

Suppose that - theoretically - FLT was false. If so, it would mean that there was a solution to a^n+b^n=c^n with the usual restrictions on the variables (I don't recall the exact restrictions offhand).

In that case, you could prove that falsity simply by providing such a solution (and explaining why it worked).

If FLT was unprovable [again, in the Gödelian sense], it would mean that there was no proof of its falsity either. But, by the previous argument, it would imply that it was not false.

Consequently, any proof of FLT's unprovability would be trivially extended into a proof of its truth. Contradiction.

[Note that this argument does not depend in any way on the existence of a proof for FLT, and indeed quite easily extends to some still-unsolved problems, such as Goldbach's conjecture.]

...anyway, the above argument was something that I was sure a smart girl from the 2180s (or indeed the 2080s) could probably quickly work out in her head, and consequently I would not have expected her to phrase the response that way. [Actually, now that I checked the actual phrasing for the first time in many years, turns out it wasn't quite as clear-cut as that, but it definitely didn't sound like "maybe it's unprovable, but if so we'll never know" either.]

So the only explanation that matched the evidence was the normal one: someone (who presumably wasn't as good at math) must have been making up the answers in her name.

[The aforementioned answer also mentioned that they did determine, at least, that Fermat was mistaken when he thought he had a proof.

My headcanon is that Fermat's proof was based on the same principles as the 1847 proof by Gabriel Lamé, and that Fermat, just like Lamé, had failed to notice the rather arcane failure of factorization which invalidated that proof.]

It's a funny and unfortunate coincidence that in S2E12 of Star Trek: TNG (aired 1989) they talk about Fermat's Last Theorem as this untractable problem that even hundreds of years into the future people are still trying to crack. Fermat wrote down the conjecture around 1637, so when the episode aired it had been an open problem for 352 years. Little did they know that Wiles would finish the proof in 1994!

[glitter-stained]

As a casual maths enthusiast I really wanna assign some of my fav characters one of my favourite maths guys' hilarious philosophies/vibes so, starting with Jason Todd:

*by which I mean he resigned from the world of academia due to disappointment in the ethical standard there, not that he thought they sucked at maths.

** as a casual enjoyer I don't know the stories and people behind most of my favourite math stuff, I'd love to see more of it!

+ Fair Warning: Again, this is very surface level and voluntarily portrayed in a comedic manner, I mean no disrespect to those guys' intelligence or their philosophy I just think they're really cool and also kinda baffling. In order, we have: Srinivasa Ramanujan, Kurt Gödel, Grigori Perelman, Richard Feynman, Ada Lovelace and Pythagoras.

Also I just noticed the typo FML 😭

[tempusedax-rerum]

All references in TiBiBiBaP

Under the cut you'll find all the little references and allusions made in Then it Becomes, it Becomes, it Becomes a Problem because I kept track of them and hey, why not, right?

It's going to include some stuff that probably seems painfully obvious. And did I miss some? Most likely, yes.

Obviously this contains MAJOR SPOILERS lol

Chapter 1:

Bill-proof suit -> The Book of Bill (TBoB from here on out)

Post-it notes -> TBoB

Shave-and-a-haircut -> classic call-and-response

“Have you come to steal my eyes?” -> S02E12, a Tale of Two Stans

Venus de Milo -> ancient Greek marble statue

Chapter 2:

“…grisly warzone that had once been their favourite taco place.” -> TBoB burrito paradox incident

“hourglass-shaped container” -> inspired by the ‘Cosmic Sand’ from S02E08 Blendin's Game and also the oracle page in Journal 3

“Vigilantes vel somniantes vel ambo…” -> this is actually a direct reference to a poem I wrote

“…you never needed the feather, Dumbo!” -> this scene in Dumbo

“The first line of the pyramid is also a theorem; it follows directly from axiom two.” -> Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page 224. All of Amorphous Shape's dialogue comes from GEB because I'm saying that all of reality is consciousness-based (specifically Axolotl-based). What is Amy? A mystery, at her core. Some kind of expression of the fractal structure of the multiverse.

"in the backroom" -> did I spell back room incorrectly?? No. I did this on purpose.

Chapter 3:

My take on the mindscape is loosely based on Plato’s theory of Forms with some scaffolded fractal panpsychism thrown in for good measure

“…grains of sand on every beach…” -> reference to Carl Sagan's book Cosmos

5D Chess with Multiverse Time Travel -> reference to the actual game

Chapter 4:

“It would be insane for a serial killer to press and stamp their own fake pain killers…” -> allusion to the Tylenol murders

Photocopier -> S01E07, Double Dipper

“Route 14” -> Journal 3

Chapter 5:

“…a large gourd with an unnervingly friendly face.” -> Journal 3

“ten distinct varieties of spectra” -> Journal 3

“a huge chess pawn” -> seen in the background of various Gravity Falls episodes (in the attic)

“Teen and Up” -> reference to AO3's rating system

“chamomile, cardamom, and dandelion root” -> chosen for their occult and medical properties: chamomile is associated with calm, cardamom is associated with love, dandelion root is associated with change. Basically a little spell to keep Ford thinking good thoughts about Bill.

Chapter 6:

“Chumbo’s hungry” -> TBoB

“singing the alphabet backwards and chanting Z-O-Z-O-Z-O” -> these are two common ouija board events that are attributed to demons

“Bring spiders! Sixer liked those.” -> TBoB

“The sum of the angles in a triangle is 180 degrees only in Euclidean geometry; it is greater in elliptic geometry, less in hyperbolic.” -> Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page 100

“party like it’s 1346!” -> this is the year the bubonic plague started

Chapter 7:

“There are these cows” -> Journal 3

“The Collector” -> a real Marvel villain

“eaten by Steve” -> Journal 3

“A mop and a bucket.” -> allusion to the various Handyman Bill AUs

“STARVING BLACK HOLES HAVE EATEN ALL LIGHT” -> that's entropy, babyyyy. Specifically, I was thinking about this video: time lapse of the future

“He wants his mother to cut the crusts off his sandwiches.” -> TBoB (burrito paradox 911 call script)

Chapter 8:

“the Purple Man” -> also a Marvel villain

“nice birthday present” -> TBoB

“I don’t want to be a fish!” -> the theory that hiccups are an old fish reflex

“Or Plato?” -> allusion to the allegory of the cave which is linked to Plato's Forms

Chapter 9:

“…has caused me harm in the following ways” -> common script for intervention letters

“A potato-shaped creature with a jelly-like consistency and four eyes” -> Journal 3

“Et tu, Brute?” -> Julius Caesar of course

“this space was originally my biospec lab” -> and that’s what it is in the show according to S02E15, The Last Mablecorn. I tried to stay true to this layout but I did make a few changes.

“He gave me rats for my birthday.” -> TBoB

Chapter 10:

“For here it is true that si paullum a summo discessit, vergit ad imum. I turned back when I saw that no man can reach the bottom of this night.” -> Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page 92

“We cannot make the loop any tighter, but we can open it wider, by choosing to insert any number of intermediate levels, such as ‘picture frame’, ‘arcade’, and ‘building…’” -> Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page 716

“Nice place to visit, but I wouldn’t want to live there.” -> direct quote from the Twilight Zone, S01E28

“The architect of my own destiny.” -> paraphrase of Jean-Paul Sartre

“Self-made man” -> paraphrase of Frederick Douglass

“portable portal device” -> allusion to the portal gun Rick and Morty... bordering on reference the further into the story we go... lol

Chapter 11:

Tachyon Queso’s Time-Loop Pizza Palazzo —> this is obviously just Chuck E. Cheez which, apparently, used to be called "Chuck E. Cheese's Pizza Time Theatre"

Chapter 12:

“Mens, corpus, in aethere separatum, spiritu unitum” –> I swear this is a reference to Supernatural but I can't remember which incantation I was thinking of

Chapter 13:

Multibear → S01E06, Dipper Vs. Manliness

"Just a couple tweaks" -> reference to Let's Get Weird by Longest Solo Ever

Chapter 14:

“If you wanted to go back and replace axiom one by its negation, you could not just do that by itself; you would have to delete all theorems which had involved axiom one in their derivations.” → Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page ?? (forgot to record the page number, so sorry lol. When I reread I'll fix this)

“Querying the system about some particular idea sets up a goal in the form of a string to be derived.” → Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page ?? (same issue as above)

“hyperdrive from crash site Omega” → Journal 3

“concentrated dark matter” → reference to Rick and Morty, S01E04, M. Night Shaym-Aliens!

“shadow realm” → reference to Yu-Gi-Oh!

“Magrathea” → planet in Hitchhiker's Guide to the Galaxy

Sikaris → planet in Star Trek (Voyager, S01E10, Prime Factors)

Naboo → planet in Star Wars

Arrakis → planet in Dune

Golden fiddle → reference to The Devil Went Down to Georgia

Chapter 15:

“Swayze-style” & “You had me at ‘ditto!’” → references to Ghost (1990)

Chapter 16:

Nothing here except a bunch of over-simplified neuroanatomy.

Chapter 17:

“Krombo-whoever-the-fuck” → allusion to Krombopulous Michael from Rick and Morty, S02E02, Mortynight Run

“she’d figured out how to set water on fire” → that one chemical that can do that. I forget. I think it's lithium? It also burns pink, so I think this was Alex's intention for Pyronica

Jheselbraum → Journal 3

Chapter 18:

“he had Jennifer Aniston beat in that regard” → reference to the Jennifer Aniston neuron

“what was his name… George? Henry?” → allusion to a series of comments where people kept getting Gary's name wrong. It really cracked me up. Like, yes, exactly! Gary is barely a person. Hilarious.

“‘Subsystem’ is just another name for an overgrown symbol, one which has gotten so complicated that it has–” → Gödel, Escher, Bach: an eternal golden braid by Hofstadter, Douglas R., page 384

“spiritui esto subiecto voluntati meae" -> and then Snooki appears! Reference to Supernatural, S9E16, Blade Runners

“Greek superstition” → parsley is associated with the dead in Greek mythology

“Yarrow? Frankincense?” & “absinthe?” → chosen for their occult properties. Yarrow is associated with removing blockages, frankincense with ritual amplification and consecration of ritual spaces, and absinthe is associated with spirit communication

Chapter 19:

“Anima, corpori fuerit corpus totem resurgent.” → reference to Supernatural, S8E1, We Need to Talk About Kevin. Fun fact: Amina (Anima backwards) just so happens to stand in for "amen" in Arabic in certain circumstances. Neat how that worked out.

And lots more oversimplified neuroanatomy. I like brain science. :3

Also thank you to my university's physics department and to some very helpful people on discord who checked my math on those three problems.

Chapter 20:

"Dammit, Janet" -> a song from Rocky Horror Picture Show

“fucking Nosferatu” → Nosferatu :) obviously :)

Chapter 21:

“Obtuse Society” → From this panel about the pitch document for TBoB (here's the actual document)

Chapter 22:

“cells, interlinked” → Pale Fire by Vladimir Nabakov

“Dr. Jacobi” → Laura Palmer's therapist in Twin Peaks

“Did the lambs get too loud for you, Clarice?” → Silence of the Lambs

“salt on Ford’s windowsills” → allusion to Supernatural. Just salt everywhere.

Chapter 23:

“stupid… calcium” → lack of calcium re-uptake is one of the causes of rigor mortis

Chapter 23:

I am purposefully drawing parallels between Bill's trauma-based dissociative experiences and my own experiences with dissociative disorders. This is also a nod to Disco Elysium!

"jelly doughnut" -> reference to the autopsy snack time trope

Chapter 24:

Nothing here

Chapter 25:

This chess game is just The Immortal Zugzwang

Chapter 26:

"a tattoo guy" -> it could be any of the embarrassing tattoos tbh (but it's definitely the "if lost return to Bill Cipher" one from TBoB, the "my muse and me" page)

Moloko plus → disgusting milk-based beverage in A Clockwork Orange

That ol’ Janx Spirit → alcoholic component of the Pan Galactic Gargle Blaster in Hitchhiker's Guide to the Galaxy

Chapter 27:

The spot in the Atlantic ocean that Ford is referencing is meant to be the Bermuda triangle

“Eyepatch?” → allusion to Theseus' Guide to Ruining a Perfectly Good Boat by @stump-not-found

The cake in this scene is an internal reference to the moment that Bill saw in chapter 7 which he was using to anchor his behaviour in subsequent chapters. In case.... anyone missed that. It was a very small moment.

"Just rebrand it as an adhesive exfoliating strip. The rash means it’s working." -> Stan's bandaid brand, "The Rip-Off," that won't give you rashes from S02E12, A Tale of Two Stans

The final scene with Bill is a reference to the one therapy session we see on thisisnotawebsitedotcom.com when you type in "even his lies are lies"

Spiders Georg -> we all know this guy, I hope