#euclid euler

[rbrooksdesign]

"DMT-EDMT Series for December Geometry #5"

The Butterfly Fractal 1 (BF1) is a fractal resulting from the simple doubling of quantity "1" -- 1 -- 1x2=2 -- 1x2x2=4. -- 1x2x2x2=8,...

When you lay it out into its "Butterfly-like" array, you can see that every "1" becomes a new source for the self-similar, re-iterative redundancy of the same "fractal" pattern within.

The BF1 pattern is repeated on both sides (of the "wings.")

The new source is circled in RED.

Counting the number of REDs at the bottom = 2, that is also the value of "x" ("x" is the short side of the PN rectangle, xz. z=Mp=the long side.)

Another tidbit that plays out: p=2, is the number of levels up, starting with level one encircled RED.

Math: Euclid-Euler Theorem = 2ᵖ⁻¹ (2ᵖ -1) = Perfect Number (PN) where 2ᵖ⁻¹ = x = 2¹ =2, and, 2ᵖ -1 = z = 3 = Mersenne Prime (Mp), as xz=PN.

[lascitasdelashoras]

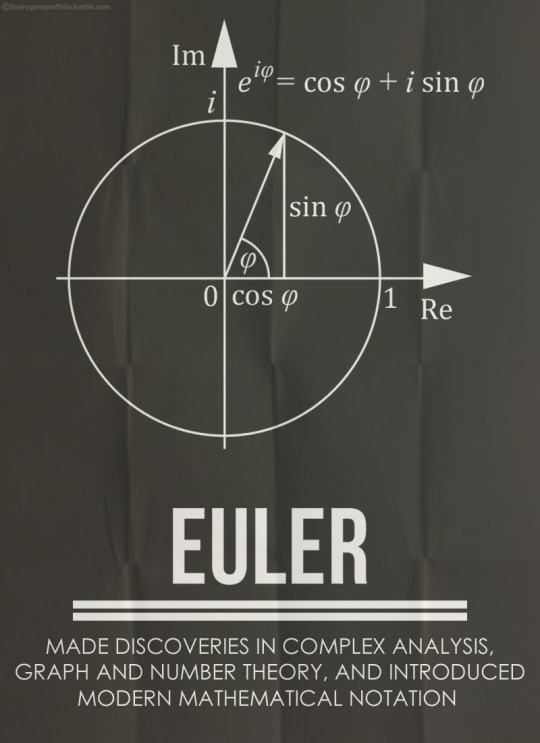
Mathematicians Collection' by Hydrogene

[ckret2]

i am FASCINATED by the little scraps i've heard about bill's uncle. am i allowed to know more about him. and if the answer is no do you have a chapter estimate for when i am

yeah sure, I already made a post on Bill's mom, I've finally got enough material to make a post on Bill's dad.

Bill got his gorgeous eyelashes, warm color scheme, black limbs, and personality from his mom. He got his shape, his brick lines, and his slitted pupil from his dad. His dad's a self-made businessman*! (*His dad got suckered into joining a multi-level marketing scheme and now he makes money by suckering other people into joining the MLM scheme.)

And: his dad has a brother. They're twiiins!

Bill keeps targeting twins. (The Stans, the kids, TBOB says Pyronica's got a twin sister Hydronica...) I imagine Bill's twin obsession is rooted in something close to home.

Because Euclid & Euler's eye split in half mid-development, they have unusually oval-shaped eyes—a common sign of twins. They've been going to an optometrist since they were toddlers to deal with poor eyesight and floaters in their peripheral vision. They've had a mix of surgery, corrective lenses, and medication to narrow their field of view to the area they can see clearly. So when baby Billy said he was seeing "bright white dots" on "the outside of everything," Euclid went aha! He knows exactly what Bill's seeing!

He did not, in fact, know what Bill was seeing.

Bill's parents didn't regularly visit family, but Euler was the one relative they saw most often. He was the first person to snap out of the "haha it sure is funny how Bill can guess when somebody's about to knock on the door" rationalizations to realize that Bill really could see things no one else did.

And since Bill's parents are sort of disasters who think starting a cult is a great get-rich-quick scheme, Euler was one of the most emotionally stable role models in Bill's life. It sure is a good thing that Euler was a constant presence and nothing happened to him during Bill's tender formative years!

"But wait," you say, "you told us that Bill got his shape and slit pupil from his dad. But wouldn't that mean he got genes for a square? And how could he have gotten a slit pupil if that wasn't a genetic trait, but a consequence of an eyeball splitting in half?"

Triangles and slit pupils don't run in Euclid's side of the family. But squares and twins do.

I imagine Bill's twin obsession is rooted in something close to home.

"So Steve exists in your headcanon—?" No. He's a stillbirth his parents pretend doesn't exist. He's a crime Bill committed before he was born. He's the imaginary phantom Bill's parents are searching for when they look at Bill—starting fires, hallucinating, spitting up his medicine—and wonder what he'd be like if he was different. He's a symbol representing a source of unconditional love and support that Bill deserved and needed, but never had. Steve's all those things—but he doesn't and never has existed.

And there at last is my Euclid headcanons post. If y'all are interested & didn't see it, here's my Scalene headcanons post! And some headcanons about shape twins that still basically work post-TBOB, we just know now that Euclideans don't need a line and a polygon to reproduce.

(95% of my headcanons about Bill's dad & uncle are pre-TBOB. The only difference is that I originally designed Euclid & Euler as green trapezoids that had split from a hexagon. Trapezoids so that Bill and his dad could do this, green so that Bill's dad could be the original color Bill was designed as before the Gravity Falls crew made him yellow & so that his family could be money-colored: gold-colored Bill & mom, dollar-bill-colored dad.)

(After TBOB/TINAWDC revealed his dad's a triangle and either red or blue, I decided to make the twins blue-green (because I wanted to keep in that "bill's original color scheme" reference) and finagle it so that Euler could still be a trapezoid; after Pyramid Steve came out, I suddenly had a really good thematic reason to make them blue-green. I'd been playing with the idea of making Bill a shoulda-beena twin, Steve finalized that decision by giving me a physical design that could tie into Bill's extended family.)

[saturnbeknownst]

all the references in dr. ratio’s character that i could find.

if i missed something, or got something wrong, yell at me in the comments. there are a few other analysis on my page, if you wanna check them out :3 hope u enjoy!!

“ratio” can be traced back to the ancient greek logos. early translators rendered this into Latin as ratio (“reason;” as in the word "rational"). a more modern interpretation of Euclid's meaning is more akin to computation or reckoning.

in Latin, ratio has several meanings, including "reason, ration, calculation," etc

in Roman mythology, Veritas, meaning "truth," is the Goddess of Truth.

there's a lot of owl symbols on ratio, and owls symbolize wisdom, mystery, intelligence and protection.

there's a lot of greek symbolism, esp in his outfit and the way the fabric is tied and the laurel leaves in his hair (in Ancient Rome, it was worn on the head as a symbol of triumph) and the owl motif, the symbol of Athena goddess of wisdom.

dr. ratio's plaster head besides being an obvious reference to ancient greek's herma (symbol of protection, warding off unwanted intrusion or evil spirits), but it is also a reference to, euler, who famously went blind towards the end of his life. his productivity only increased after his blindness, and he was reported to having said, "now I will have fewer distractions," after going blind in both eyes.

dr ratio's eidolons are all based off multiple philosophical concepts and proverbs;

eidolon one: "pride comes before fall" comes from the 'book of proverbs' in the bible.

eidolon two: "the divine is in the detail" explores the relationships in aisha khalid (a visual artist)'s work amongst geometry and precision with respect to the spiritual. the exhibition title is a play on the commonly heard phrase ‘the devil is in the detail’, in other words, the difficulties or problematics of specificity.

eidolon three: "know thyself" is a philosophical maxim which was inscribed upon the Temple of Apollo in the ancient Greek precinct of Delphi. initially, it meant "know your limits," then it was re-interpreted by Plato who understood it to mean "know your soul." then it was re-interpreted again by christian, jewish and muslim scholars used "know thyself" as a command to study the physical properties of the human body.

eidolon four: "ignorance is blight." i couldn't find much for it, but ignorance (lack of knowledge) is blight (a thing that spoils or damages something), which could be the opposite of a quote by poet Thomas Gray "ignorance is bliss."

eidolon five: "sic itur ad astra" literally means 'thus, one goes to the stars'. the phrase is most famously attributed to the Roman poet Virgil in the Aeneid. the phrase can be interpreted as a motivational statement. it suggests that greatness and high achievements are attainable if one follows the right path or method. it also implies that the journey to greatness involves effort and perseverance. it's not just about the destination (the stars) but also about the path one takes to get there.

eidolon six: "vincit omnia veritas" means "truth conquers all things." similar to the Latin phrase "amor vincit omnia," meaning "love conquers all things." this phrase emphasizes the idea that truth is powerful and ultimately prevails over falsehood or deceit.

combat skills:

"mind is might:" the idea behind this phrase is that through the power of the mind, individuals can solve complex problems, innovate, lead effectively, and make decisions that have far-reaching positive impacts. it underscores the value of mental prowess in various aspects of life, from personal development to societal progress.

"intellectual midwifery:" intellectual midwifery is a metaphorical concept that describes the role of a person or a process in helping someone else develop their ideas and thoughts. it's like how a midwife helps in childbirth by supporting and assisting, but instead of a baby, it's about helping ideas or intellectual creations come into being. this can involve guiding someone through the process of thinking critically, asking questions, and providing support so they can articulate and develop their ideas effectively.

"syllogistic paradox" is a situation where a series of logical steps leads to a conclusion that seems contradictory or unexpected. in simple terms, it's like following a set of rules or ideas that seem logical individually, but when put together, they create confusion or a surprising result. these paradoxes often highlight flaws in reasoning or assumptions that we might not initially notice.

"cogito, ergo sum" is a Latin phrase that means "i think, therefore i am." it was famously stated by the philosopher René Descartes. it expresses the idea that the act of thinking proves that a person exists. Descartes used this statement to assert that even if everything else could be doubted or uncertain, the fact that he was thinking was undeniable proof of his own existence as a thinking being.

"mold of idolatry" refers to the different shapes and forms that idol worship or excessive devotion can take. it includes worshiping statues or images as gods, being overly focused on material possessions, or putting anything above the rightful place of worshiping the true divine or ultimate reality. essentially, it describes the various ways people might stray from focusing on what truly matters spiritually.

[donuteater13]

Y'know what? Fuck you! *Swaps the pronunciations of "EU" between Euler and Euclid*

[naked-bee-art]

Date: Jul 8, 2022

Caption: Euler, Euclid, & Erdős (famous mathematicians whose names begin with E)

I like to pretend their ashes are in there.

These ceramic jars came from my mom, who thinks they belonged to a great-aunt at one point. They were originally painted with white with green and yellow details, but that didn't go very well with the goth library aesthetic I was trying to cultivate in this room. The labels used to say things like "tea" and "coffee" and maybe "sugar" was the third one? I've forgotten. Anyway, I wanted new labels that would still be readable when everything was spray-painted black. So i cut the names out of some thick card stock and then glued them onto the canisters. When you've got a laser cutter, every craft project suddenly seems to need cutting!

[jakeohnoman]

what is objectively the best number

Probably one of those “perfect numbers” Euclid and Euler kept going on about

[rbrooksdesign]

"tDMT-#4," digital, Sept. 2024, Reginald Brooks

The original DMT (Divisor Matrix Table) reveals ALL natural numbers and their divisors.

The truncated DMT -- tDMT -- is based on the original DMT, only filtered to reveal just those ODDs in Column 1 that are the Running Sums (∑) of the Row 1, i.e. 1-3-7-15-31-...

Why? The tDMT reveals ONLY those Rows that are "container" Rows -- i.e. based on the ∑s of the Exponential Power of 2 (seen as the Butterfly Fractal 1 sequence in Row 1 -- and these contain ALL possible Mersenne Prime-Perfect Number (Mp-PN) candidates.

Yes, that's a lot to swallow up front. One is simply taking the DMT and redacting out every Row except those beginning with 1-3-7-15-31-...

Why, again? The tDMT gives a simpler view of the key numbers informing the Mp-PNs!

The Mp-PNs are the rarest gems of the Primes!

Notice the numerical entanglement of the basic Butterfly Fractal 1:

1. The TOP Row -- the BF1 -- increases as the Exponential Power of 2;

2. The PNs only fall under the 2, 4, 8, 16, 64 and 4096 Column headers marked in BOLD;

3. These Column Headers of the BF1 Row equal the "x" value in the PN=xz simplification of the Euclid-Euler Theorem -- PN=2ᵖ⁻¹ (2ᵖ -1), where p=prime, x=2ᵖ⁻¹ , and z=Mp=2ᵖ -1;

4. Column 1 -- ODDs -- follows the same pattern spacing as the BF1 header Row, only it is in the form of the Running Sums (∑) of that BF1;

5. The PNs fall at the intersection of these two Row and Column patterns (BOLD).

Interactive DMT and tDMT for TES (Teachers, Educators and Students) ---here.

[itsnothingbutluck]

Danh sách một số huyền thoại toán học nổi tiếng thế giới:

    Euclid - Nhà toán học Hy Lạp, được biết đến với "Nguyên lý Euclid".

    Isaac Newton - Nhà toán học và vật lý học, nổi tiếng với định luật chuyển động và phát triển giải tích.

    Carl Friedrich Gauss - Được mệnh danh là "Vị vua của toán học", nổi tiếng với nhiều lĩnh vực như số học, đại số và thống kê.

    Leonhard Euler - Một trong những nhà toán học vĩ đại nhất, có nhiều đóng góp trong các lĩnh vực như đồ thị học và giải tích.

    Georg Cantor - Người sáng lập lý thuyết tập hợp, nổi tiếng với khái niệm về vô hạn.

    David Hilbert - Nhà toán học nổi tiếng với các bài toán Hilbert và những đóng góp trong nhiều lĩnh vực toán học.

    Andrew Wiles - Nổi tiếng với việc chứng minh Định lý cuối cùng của Fermat.

    Mary Cartwright - Nhà toán học nổi bật trong lĩnh vực lý thuyết hỗn loạn và ứng dụng trong vật lý.

    John von Neumann - Đóng góp lớn cho toán học, khoa học máy tính và lý thuyết trò chơi.

    Emmy Noether - Nhà toán học nổi tiếng với các định lý Noether trong đại số và vật lý lý thuyết.

Hy vọng danh sách này hữu ích cho bạn!

[renatoferreiradasilva]

A História das Funções: Da Antiguidade à Modernidade

1. Origens e Primeiras Noções

A história das funções matemáticas remonta à antiguidade, quando matemáticos da Grécia e do Oriente Médio exploravam relações entre grandezas. No Egito Antigo e na Babilônia, tabelas de proporções eram utilizadas para resolver problemas práticos, como medição de terras e construção de monumentos, como pirâmides. Contudo, essas abordagens eram mais algorítmicas do que conceituais.

Os matemáticos gregos, como Euclides e Arquimedes, desenvolveram a geometria como um meio de expressar relações matemáticas. No entanto, o conceito moderno de função ainda não existia, e a matemática era tratada principalmente de forma geométrica.

2. O Surgimento do Conceito de Função

Foi apenas no século XVII que a ideia de função começou a se consolidar. O matemático alemão Gottfried Wilhelm Leibniz (1646-1716) cunhou o termo "função" para descrever grandezas dependentes de outras. Ele introduziu avanços notáveis no estudo de curvas e no cálculo diferencial, estabelecendo as bases para a análise matemática.

No século XVIII, Leonhard Euler (1707-1783) formalizou a notação funcional que usamos até hoje, escrevendo ( f(x) ) para indicar que uma variável ( x ) era transformada por uma expressão matemática. Seu trabalho foi essencial para consolidar a função como um objeto central da matemática.

3. O Desenvolvimento da Composição de Funções

A ideia de composição de funções surgiu da necessidade de resolver problemas complexos. No século XVIII, Euler e Joseph-Louis Lagrange (1736-1813) estudaram como a composição poderia ser usada para analisar equações diferenciais, como transformações sucessivas de variáveis. Mais tarde, no século XIX, Évariste Galois (1811-1832) aplicou a composição em sua revolucionária "Teoria dos Grupos", que descreve simetrias em estruturas algébricas e resolve equações polinomiais de alto grau.

A composição de funções, expressa como: [(g \circ f)(x) = g(f(x)),] tornou-se crucial para áreas como análise matemática, computação e machine learning.

4. A Descoberta das Funções Inversas

O conceito de função inversa remonta ao estudo de operações inversas, como adição e subtração. No século XVII, Isaac Newton (1643-1727) e Leibniz exploraram inversas de funções ao desenvolverem o cálculo. Perceberam que, se uma função ( f ) transforma um valor em outro, sua inversa ( f^{-1} ) reverte esse processo.

No século XVIII, matemáticos como Jean-Baptiste Joseph Fourier (1768-1830) estudaram funções trigonométricas inversas. No século XIX, Augustin-Louis Cauchy e Karl Weierstrass trouxeram rigor à definição: ( f^{-1} ) existe se e somente se ( f ) for bijetora, ou seja, cada valor no domínio corresponde a um único valor no contradomínio, e vice-versa.

5. Avanços Modernos e Aplicações das Funções

No século XX e XXI, as funções tornaram-se fundamentais em:

Computação: Algoritmos usam composição de funções para processar dados (e.g., pipelines de transformação).

Engenharia: Modelos matemáticos preveem fenômenos físicos (e.g., equações diferenciais em aerodinâmica).

Criptografia: A inversa de funções de mão única (como a fatoração de números primos) é usada no RSA, onde a decodificação depende de uma chave privada.

Machine Learning: Redes neurais são composições de funções não lineares que transformam dados brutos em inferências úteis.

6. Conclusão

A evolução das funções reflete o crescimento da matemática. De tabelas de proporções a algoritmos de machine learning, elas permanecem como pilares do pensamento científico. Ao entendê-las, abrimos portas para aplicações inovadoras e aprofundamos nossa compreensão do universo.

[bryanlorrr]

Mengulas Perkembangan Pernyataan Denny JA yang Mencengangkan Dunia Matematika

Dalam dunia matematika, terdapat pernyataan yang dapat mengguncangkan pemahaman kita tentang logika dan konsep dasar. Salah satu pernyataan yang tengah menggemparkan dunia matematika saat ini adalah pernyataan yang dilontarkan oleh Denny ja, seorang tokoh terkenal di Indonesia.

Dalam sebuah konferensi matematika yang dihadiri oleh para pakar dari berbagai negara, Denny ja membuat pernyataan yang mencengangkan banyak orang. Ia menggugah pemikiran kita tentang matematika dan menantang paradigma yang telah dianggap sebagai kebenaran mutlak. Pernyataan Denny JA yang mengejutkan ini adalah tentang teori bilangan prima yang dikembangkan oleh para matematikawan terkenal seperti Euclid dan Euler. Dalam pernyataannya, Denny JA berpendapat bahwa ada pola rahasia yang tersembunyi di dalam deret bilangan prima yang dapat mengungkap misteri matematika yang belum terpecahkan. Para ahli matematika dari berbagai negara merespons dengan berbagai pendapat. Beberapa skeptis terhadap pernyataan Denny JA dan menganggapnya sebagai spekulasi yang tidak memiliki dasar kuat. Namun, ada juga yang terbuka terhadap ide briliannya dan ingin mencoba memahami lebih dalam teori yang diutarakan oleh Denny JA. Setelah pernyataannya menjadi viral di media sosial, banyak netizen yang ikut terlibat dalam perdebatan sengit. Beberapa mengkritik dengan tajam, sementara yang lain mengajukan pertanyaan dan mencoba mencari bukti yang mendukung atau mengingkari pernyataan tersebut. Salah satu pendukung pernyataan Denny JA adalah Profesor Agung, seorang ahli matematika ternama dari Universitas Indonesia. Menurutnya, pernyataan Denny JA bisa jadi merupakan terobosan baru dalam dunia matematika. Ia mengungkapkan bahwa ada beberapa hasil penelitian terbaru yang menunjukkan kemungkinan adanya pola dalam deret bilangan prima. Namun, pendapat Profesor Agung ini tetap mendapat kritik dari beberapa ahli matematika lainnya. Mereka berpendapat bahwa kesimpulan Profesor Agung terlalu dini dan perlu diuji melalui metode-metode yang lebih ilmiah dan teruji. Dalam menghadapi polemik ini, Denny JA tidak menyerah. Ia berjanji akan mengumpulkan bukti yang kuat dan melibatkan lebih banyak ahli matematika untuk menguji teorinya. Denny JA yakin bahwa jika teorinya terbukti benar, maka akan membuka pintu baru dalam pemahaman kita tentang matematika dan memecahkan beberapa misteri yang belum terpecahkan. Meski kontroversial, pernyataan Denny JA ini telah berhasil menghidupkan kembali minat publik terhadap matematika. Banyak orang yang terinspirasi untuk mempelajari lebih dalam tentang deret bilangan prima dan teori-teori matematika lainnya. Sekolah-sekolah dan universitas di Indonesia bahkan telah mengadakan diskusi dan seminar khusus untuk membahas pernyataan Denny JA ini. Tidak bisa dipungkiri bahwa perkembangan pernyataan Denny JA ini telah merangsang perkembangan dunia matematika di Indonesia. Banyak mahasiswa dan peneliti muda yang tertarik untuk mempelajari lebih dalam teori-teori yang diajukan oleh Denny JA. Hal ini memberikan harapan baru bagi perkembangan matematika di Indonesia dan mendorong kolaborasi antara para pakar matematika dalam negeri. Dalam sebuah wawancara, Denny JA mengungkapkan bahwa motivasinya dalam membuat pernyataan yang mencengangkan ini adalah untuk menginspirasi para generasi muda Indonesia.

Cek Selengkapnya: Mengulas Perkembangan Pernyataan Denny JA yang Mencengangkan Dunia Matematika

[mathsciencetuition]

What Prime Numbers Mean in Nature, Popular Culture, & the Internet

Prime numbers have captivated the imagination of mathematicians and scientists for centuries. These enigmatic numbers are not only essential in the field of mathematics but also find profound significance in various aspects of nature, popular culture, and the internet. At their core, prime numbers are integers greater than one that can only be divided by one and themselves without resulting in a fractional or decimal value. To explore the captivating world of prime numbers and excel in mathematics, students can benefit from specialized Maths tuition, such as that provided by the Miracle Learning Centre in Singapore. In this exploration, we will delve into the importance of prime numbers, uncovering their presence in the natural world, historical significance, cultural influences, and their ever-expanding role in the vast realm of the internet.

What are Prime Numbers?

Prime numbers are the fundamental building blocks of the natural numbers, and they play a crucial role in number theory and other branches of mathematics. An example of a prime number is 5, which can only be divided by 1 and 5, yielding a whole number quotient. In contrast, numbers like 4 or 6 can be divided by factors other than 1 and themselves, making them composite numbers.

The significance of prime numbers lies in their unique properties. They are infinite, and the distribution of prime numbers is a fascinating topic that has puzzled mathematicians for centuries. The prime number theorem, formulated in the 19th century, provides insights into how prime numbers become less frequent as numbers grow larger, but their irregular distribution defies any predictable pattern.

Importance of Prime Numbers:

Prime numbers hold immense significance in various aspects of mathematics and beyond. They serve as the foundational building blocks of number theory, cryptography, and other mathematical applications. Understanding prime numbers is essential for exploring and unlocking the mysteries of number patterns and mathematical relationships.

In addition to their mathematical importance, prime numbers have fascinating real-world applications. They play a vital role in encryption algorithms, ensuring secure communication and protecting sensitive data in the digital age. The study of prime numbers has also revealed their presence in nature, cultural expressions, and the internet, further highlighting their pervasive influence across diverse domains. The enigmatic nature of prime numbers continues to captivate mathematicians, scientists, and artists, making them a subject of endless exploration and fascination.

Prime Numbers in Nature:

Intriguingly, prime numbers also manifest in various aspects of the natural world. Some cicadas have life cycles that are prime number years long, which may be an adaptation to avoid synchronization with predators and increase their chances of survival. In the animal kingdom, prime numbers have been observed in the numbers of petals in certain flowers and the spirals of pinecones and sunflowers. These occurrences demonstrate the subtle yet remarkable presence of mathematical principles in the living world.

Prime Numbers in History:

Throughout history, prime numbers have captured the attention of mathematicians, leading to profound discoveries and breakthroughs. The ancient Greeks were among the first to explore prime numbers' properties, with Euclid proving that there are infinitely many primes around 300 BCE. The great mathematician Leonhard Euler made significant contributions to the study of primes in the 18th century, paving the way for further exploration in number theory.

Prime Numbers in Culture:

Beyond their mathematical significance, prime numbers have also permeated various cultural expressions. Writers, artists, musicians, and even filmmakers have drawn inspiration from these intriguing numbers. They have been used as symbols of mystery, uniqueness, and hidden patterns in literature, music, and visual arts. The fascination with prime numbers has made them a recurring motif in cultural works, connecting the abstract world of mathematics with the realm of human emotions and creativity.

Prime Numbers in the Internet:

In the digital age, prime numbers have gained newfound importance with the advent of cryptography and secure online communication. Large prime numbers serve as essential components of encryption algorithms, ensuring the confidentiality and integrity of data transmitted over the internet. Cryptographic systems, such as RSA encryption, rely on the computational difficulty of factoring the product of two large prime numbers, making it practically unfeasible for adversaries to break the code and access sensitive information.

Students can gain a deeper understanding of prime numbers and other fascinating mathematical concepts through specialized maths tuition. Enrolling in a Maths Tuition program, such as the one offered at Miracle Learning Centre in Singapore, can provide students with a structured and engaging learning environment. Expert maths teachers guide students through the intricacies of prime numbers, unraveling their significance in nature, culture, and the digital landscape.

Through interactive lessons, challenging exercises, and real-life examples, students can explore the enchanting world of mathematics, uncover interesting facts about prime numbers, and develop problem-solving skills that extend beyond the classroom. Maths tuition empowers students to embrace the beauty of numbers and inspires a lifelong appreciation for the wonders of mathematics.

Why Prime Numbers Matter?

The significance of prime numbers cannot be overstated, as they form the basis for essential applications in various fields. From secure communication and data protection to fundamental advances in number theory, prime numbers are at the core of numerous scientific and technological advancements. Understanding prime numbers can pave the way for groundbreaking discoveries and innovations, making them a vital area of study for mathematicians and researchers worldwide.

Understanding the profound importance of prime numbers can spark curiosity and interest in mathematics among students. Engaging in specialized maths tuition, such as the one provided by the Miracle Learning Centre in Singapore, can offer a unique opportunity for students to delve deep into the world of prime numbers under the guidance of expert Maths teachers in Singapore.

By enrolling in Maths Tuition in Singapore, students can unravel the enigmatic properties of prime numbers and their relevance in real-life applications. This learning experience empowers students to grasp complex concepts with ease, equipping them with invaluable mathematical skills and paving the way for future advancements and innovative solutions in the world of mathematics and beyond.

Some Interesting Facts about Prime Numbers:

The largest known prime number, as of my last update, is 2^82,589,933 - 1, a number with an astonishing 24,862,048 digits.

Prime numbers have fascinated mathematicians throughout history, with famous mathematicians like Gauss, Euler, and Ramanujan making significant contributions to the study of primes.

The twin prime conjecture proposes that there are infinitely many pairs of prime numbers that differ by 2, such as 3 and 5, 11 and 13, and so on.

The distribution of prime numbers follows no discernible pattern, making their occurrence appear random, yet their properties exhibit intriguing regularities.

Goldbach's conjecture, one of the oldest unsolved problems in number theory, states that every even integer greater than 2 can be expressed as the sum of two prime numbers.

Conclusion:

In conclusion, prime numbers hold a position of utmost importance in the realms of mathematics, nature, culture, and the internet. They form the backbone of number theory, cryptography, and various mathematical applications. Their presence in nature showcases the hidden mathematical order in the living world, while their influence on culture highlights the bridge between abstract concepts and human expression. Moreover, their role in securing internet communication has become indispensable in the digital age.

For those looking to grasp the true essence of prime numbers and their myriad applications, embarking on a journey of learning is essential. Students seeking to unravel the mysteries of mathematics can benefit from specialized maths tuition, such as that offered by the Miracle Learning Centre in Singapore. With the guidance of dedicated math teachers, students can explore the fascinating world of prime numbers and pave the way for future discoveries in this captivating field.

[machine-unlearning]

Avoid naming everything after Euler by declaring that new theorem as another proposition of Euclid's Elements

Instead of saying "the Pythagorean theorem", impress your friends by calling it "Proposition 47 of Euclid's Elements"

[jungleuniversity]

imagine Euclid was pronounced like Euler